22.8: Додаток B
- Page ID
- 76139
Рішення різних проблем
За пропорціями,\( \frac{h}{k}=\frac{n}{x}\)\( \frac{h}{l}=\frac{m}{x}\) і тому\( \frac{h}{k}+\frac{h}{l}=1\).
Тому Піфагор:
\( h\left(\frac{1}{\sqrt{a^{2}-x^{2}}}+\frac{1}{\sqrt{b^{2}-x^{2}}}\right)=1.\).
Все, але\( x\) відомо в цьому рівнянні, яке тому можна вирішити для\( x\). Є кілька способів її вирішення; ось пропозиція. Якщо поставити цифри, рівняння стає
\( 3\left(\frac{1}{\sqrt{64-x^{2}}}+\frac{1}{\sqrt{100-x^{2}}}\right)-1=0.\)
Покладіть\( X= 100-x^{2}\), і рівняння стає
\( 3\left(\frac{1}{\sqrt{X-36}}+\frac{1}{\sqrt{X}}\right)-1=0.\)
Це може бути написано\( f(X)=3(A+B)-1=0\), де\( A\) і\( B\) знаходяться очевидні функції\( X\). Диференціація по відношенню до\( X\) дає\( f'(X)=-\frac{3}{2}(A^{3}+B^{3})\) і Ньютона-Рафсона ітерація\( (X=X-\frac{f}{f'})\) незабаром дає\( X\), з якої потім з'ясовується, що\( x\) = 6,326 182 м.
У кообертається рамі боб знаходиться в рівновазі під дією трьох сил — своєї ваги, натягу в струні і відцентрової сили. (Якщо вам не подобаються обертові опорні рами і відцентрова сила, вам буде легко зробити це «належним чином».) Вирішіть сили перпендикулярно струні:\( ml_{0}\sin\alpha\Omega^{2}.\cos\alpha\ =\ mg\sin\alpha\) і завдання закінчено.
Підйом або опускання дошки не застосовує жодних крутних моментів до системи, тому кутовий момент\( L\) зберігається. Тобто,
\[ L=ml^{2}\sin^{2}\theta.\omega \qquad \text{is constant.} \tag{1}\label{1} \]
У нас також є, що
\[ \text{g}\ =\ l\cos\theta.\omega. \tag{2}\label{2} \]
i. виключити w з цих рівнянь. Це дає:
\[ l^{3}\sin^{3}\theta\tan\theta=\frac{L^{2}}{\text{g}m^{2}} \tag{3}\label{3} \]
який є постійним.
II. Виключити\( l\) з рівнянь (\( \ref{1}\)) і (\( \ref{2}\)). Це дає:
\[ \omega^{3}\cot^{2}\theta=\frac{m\text{g}^{2}}{L}, \tag{4}\label{4} \]
який є постійним.
iii. Виключити\( \theta\) з рівнянь (\( \ref{1}\)) і (\( \ref{2}\)). Це дає:
\[ \omega^{3}\left(\omega l^{2}-\frac{L}{m}\right)=\text{g}^{2}. \tag{5}\label{5} \]
(Перевірте розміри всіх рівнянь.) Тоді ми можемо отримати\( \frac{L}{m}\) від рівняння (\( \ref{1}\)) і, отже,
\( \omega^{3}(\omega l^{2}-\Omega l_{0}^{2}\sin^{2}\alpha)=\text{g}^{2}\),
який є постійним.
\( l^{3}\sin^{3}\theta\tan\theta=l_{0}^{3}\sin^{3}\alpha\tan\alpha=0.023675\ \text{m}^{3}.\)
Хоча нас просять побудувати графік\( \theta\) вертикально проти\( l\) горизонталі, простіше при розробці числових значень обчислити\( l\) як функцію\( \theta\). Тобто,
\( l\ =\ \frac{0.287142}{\sin\theta\sqrt[3]{\tan\theta}}\).
(Число в чисельнику - кубічний корінь 0.023675.)
Для\( l\) = 40 см = 0,4 м напіввертикальний кут задається
\( \sin^{3}\theta\tan\theta=0.369\ 923\).
Рішенням цього є
\( \theta=45^{\circ}\ 31'\).
(Див. Розділ 1.4 Небесної механіки, якщо вам потрібно знати, як вирішити рівняння\( f(x)=0\).)
\( \omega^{3}\cot^{2}\theta=\Omega^{3}\cot^{2}\alpha\)
З наведеними даними це і є\( \omega^{3}=199.385\tan^{2}\theta\).
iii. \( \omega^{3}(\omega l^{2}-\Omega l_{0}^{2}\sin^{2}\alpha)=\ \Omega^{3}(\Omega l_{0}^{2}\ -\ \Omega l_{0}^{2}\sin^{2}\alpha)=\Omega^{4}l_{0}^{2}\cos^{2}\alpha\).
Тобто,\( \omega^{3}(\omega l^{2}\ -a)=\ \text{g}^{2}\) де, з наведеними вихідними даними,
\( a=0.48168\ \text{m}^{2}\text{s}^{-1}\)і\( \text{g}^{2}\ =\ 96.04\ \text{m}^{2}\text{s}^{-4}\).
Хоча нас просять побудувати графік\( \omega\) вертикально проти\( l\) горизонталі, легше при відпрацюванні числових значень обчислити l як функцію\( \omega\). Тобто,
\( l^{2}\ =\ \frac{\text{g}^{2}}{\omega^{4}}+\frac{a}{\omega}\).
Вирішити вищевказане рівняння для\( \omega\) може бути трохи простіше з заміною\( u\) for\( \frac{1}{\omega}\):
\( \text{g}^{2}u^{4}\ +\ au\ -l^{2}=0\).
При \( l\)= 0,6 м це дає\( u\) = 0,226121 рад - 1 с, а значить\( \omega\) = 4,422 рад с - 1. Як і в частині (b) i, необхідно знати, як вирішити рівняння\( f(x)=0\). Див. Розділ 1.4 Небесної механіки, якщо вам потрібно знати, як це зробити.
Горизонтальних зусиль немає, тому що стіл гладкий. Тому центр маси стрижня падає вертикально. Центр маси стрижня падає вертикально.
З енергетичних міркувань
\[ \frac{1}{2}m\dot{y}^{2}\ +\ \frac{1}{2}\left(\frac{1}{3}ml^{2}\right)\dot{\theta}^{2}\ +\ mgy\ = \text{constant}. \tag{1}\label{4.1} \]
Але\( y\ =\ l\cos\theta\) і тому\( \dot{y}=-l\sin\theta.\dot{\theta}\).
\[ (3\sin^{2}\theta\ +\ 1)l\dot{\theta}^{2}\ +\ 6\text{g}\sin\theta=C. \tag{2}\label{4.2} \]
Спочатку\( \theta=\dot{\theta}=0, \quad \therefore C=6\text{g}\).
\[ \underline{\underline{\dot{\theta}^{2}\ =\ \frac{6\text{g}(1-\cos\theta)}{l(3\sin^{2}\theta+1)}}} \tag{3}\label{4.3} \]
Крім того, так як\( \dot{y}^{2}=l^{2}\sin^{2}\theta\dot{\theta}^{2}\) і\( \dot{x}^{2}=l^{2}\cos^{2}\theta\dot{\theta}^{2}\), ми отримуємо
\[ \underline{\underline{\dot{y}^{2}\ =\ \frac{6\text{g}l\sin^{2}\theta(1-\cos\theta)}{3\sin^{2}\theta+1}}} \tag{4}\label{4.4} \]
і
\[ \underline{\underline{\dot{x}^{2}\ =\ \frac{6\text{g}l\cos^{2}\theta(1-\cos\theta)}{3\sin^{2}\theta+1}}} \tag{5}\label{4.5} \]
Звичайно\( \dot{\theta}\) і монотонно\( \dot{y}\) збільшуватися з\( \theta\); але\( \dot{x}\) починається і закінчується з нуля, і повинен пройти максимум. З\( c\ =\ \cos\theta\), Рівняння (\( \ref{5}\)) може бути записано
\[ \dot{x}^{2}\ =\ \frac{6\text{g}lc(1-c)}{4-3c^{2}}, \tag{6}\label{4.6} \]
і\( \dot{x}^{2}\) диференціюючи по відношенню до\( c\), ми бачимо, що\( \dot{x}^{2}\) найбільший під кутом,\( \theta\) заданим
\[ 3c^{2}\ -12c+8=0, \tag{7}\label{7} \]
рішення якого є\( \underline{\underline{\theta\ =\ 37^{\circ}\ 50'}}\).
Якщо довжина стрижня дорівнює 1 м (\( l\)= 0,5 м) і\( \dot{x}\) = 1 м с - 1, рівняння\( \ref{4}\) стає
\[ 26.4c^{2}\ -29.4c\ +\ 4\ =\ 0, \tag{8}\label{8} \]
і два рішення -\( \underline{\underline{\theta\ =\ 17^{\circ}\ 15'}}\) і\( \underline{\underline{80^{\circ}\ 52'}}\).
Читач, який зробив усі проблеми до цих пір, буде усвідомлювати важливість можливості миттєво вирішити рівняння\( f(x)=0\). Якщо ви ще цього не зробили, вам слід написати програму для комп'ютера або калькулятора, яка дозволяє зробити це миттєво і в один момент. Див. Розділ 1.4 Небесної механіки, якщо вам потрібно знати, як це зробити.
Якщо ви хочете знайти нормальну\( N\) реакцію столу на нижньому кінці стрижня, ви можете почати з вертикального рівняння руху\( m\ddot{y}=N-mg\). Диференціювати рівняння (\( \ref{4}\)):\( 2\dot{y}\ddot{y}=\) що завгодно, і використовувати рівняння
\( \ref{4}\)знову для\( \dot{y}\). Це виглядає як досить важка і нецікава алгебра для мене, тому я не буду її переслідувати. Може бути кращий спосіб...
На малюнку нижче я позначив червоним кольором сили на стрижень, а саме його вага\( mg\) і горизонтальну і вертикальну складові\( X\) і\( Y\) реакцію шарніра на стрижень. Я також позначив зеленим кольором поперечну та радіальну складові прискорення центру маси. Поперечна складова є,\( l\ddot{\theta}\) а радіальна складова - доцентрове прискорення\( l\dot{\theta}^{2}\) .центр маси. Поперечна складова є,\( l\ddot{\theta}\) а радіальна складова - доцентрове прискорення\( l\dot{\theta}^{2}\).
З розгляду моменту сили\( mg\) про нижній кінець стрижня видно, що кутове прискорення є
\[ \ddot{\theta}=\frac{3\text{g}\sin\theta}{4l} \tag{1}\label{5.1} \]
і записуючи\( \ddot{\theta}\) як\( \frac{\dot{\theta}d\dot{\theta}}{d\theta}\) і інтегруючи (з початковими умовами\( \theta\ =\ \dot{\theta}\ =\ 0\)), або з енергетичних міркувань, отримуємо кутову швидкість:
\[ \dot{\theta}^{2}=\frac{3\text{g}(1-\cos\theta)}{2l}. \tag{2}\label{5.2} \]
Горизонтальні і вертикальні рівняння руху бувають:
\[ X=ml(\ddot{\theta}\cos\theta-\dot{\theta}^{2}\sin\theta) \tag{3}\label{5.3} \]
і
\[ mg\ -\ Y\ =\ ml(\ddot{\theta}\sin\theta\ +\ \dot{\theta}^{2}\cos\theta). \tag{4}\label{5.4} \]
(Як ніколи, перевірте розміри - і порахуйте точки!)
Після підміни на\( \ddot{\theta}\) і\( \dot{\theta}^{2}\) знаходимо
\[ X\ =\ \frac{3}{4}m\text{g}\sin\theta(3\sin\theta-2) \tag{5}\label{5.5} \]
і
\[ Y\ =\ \frac{1}{4}m\text{g}(1-3\cos\theta)^{2}. \tag{6}\label{5.6} \]
Результати слідують негайно.
Назвіть довжину стрижня\( 2l\). Спочатку висота над таблицею її центру мас дорівнює\(l \cos 40\) °, а її гравітаційна потенційна енергія -\( m\text{g}\ l\ \cos40^{\circ}\). Коли він потрапляє на стіл з кутовою швидкістю w, його кінетична енергія дорівнює\( \frac{1}{2}I\omega^{2}\ =\ \frac{1}{2}\left(\frac{4}{3}ml^{2}\right)\omega^{2}\ =\ \frac{2}{3}ml^{2}\omega^{2}\). Тому,
\( \omega\ =\ \sqrt{\frac{3\text{g}\cos40^{\circ}}{2l}}\ =\ \underline{\underline{4.746\ \text{rad s}^{-1}\ =271.9\ \text{deg s}^{-1}}}\).
Щоб знайти витрачений час, ви можете використовувати Equation 9.2.10:
\[ t\ =\ \sqrt{\frac{I}{2}}\int_{40^{\circ}}^{90^{\circ}}\frac{d\theta}{\sqrt{E-V(\theta)}}. \tag{7}\label{6.7} \]
Ось,\( I\ =\ \frac{3}{4}ml^{2},\ E=m\text{g}l\cos40^{\circ},\ V(\theta)\ =\ m\text{g}l\cos\theta\) а значить
\[ t\ =\ \sqrt{\frac{2l}{3\text{g}}}\int_{40}^{90}\frac{d\theta}{\sqrt{\cos40^{\circ}-\cos\theta}}. \tag{8}\label{6.8} \]
Величина величини перед знаком інтеграла дорівнює 0,184428 с Щоб знайти значення інтеграла потрібно або бути експертом в еліптичних інтегралах, або (швидше за все і корисніше), щоб ви знали, як інтегрувати чисельно (див. Небесна механіка 1.2). Я роблю значення інтеграла 2,187314, щоб час взятого дорівнювало 0,4034 секунди. При інтеграції зверніть увагу, що значення integrand нескінченно на нижній межі. Як боротися з цією складністю, розбирається в Небесній Механіці 1.2. Він не може бути заглянутий.
Ось схема. Сили - це вага\( m\text{g}\) стрижня, і сила столу на штоку. Однак я вирішила останні на дві складові — нормальна\( N\) реакція столу на стрижень, і сила тертя\( F\), яка може бути або вліво, або вправо, залежно від того, стрижень має тенденцію ковзати вправо або вліво. \( F\)Величина менше, ніж до тих\( \mu N\) пір, поки стрижень не тільки про ковзання. Коли стрижень ось-ось ковзає,\( F=\mu N,\ \mu\) будучи коефіцієнтом граничного статичного тертя.
Так само, як і в задачі 5, рівняння руху, поки стрижень не ковзає, є
\[ F\ =\ \frac{3}{4}m\text{g}\sin\theta(3\cos\theta-2) \tag{1}\label{7.1} \]
і
\[ N\ =\ \frac{1}{4}m\text{g}(1-3\cos\theta)^{2}. \tag{2}\label{7.2} \]
\[ \frac{F}{N}=\frac{3\sin\theta(3\cos\theta-2)}{(1-3\cos\theta)^{2}}. \tag{3}\label{7.3} \]
На малюнку нижче показано\( \frac{F}{N}\) як функцію\( \theta\). Один бачить, що, як стрижень падає,\( \frac{F}{N}\) збільшується, і, як тільки він досягає значення\( \mu\), стрижень буде ковзати. Однак ми бачимо, що\( \frac{F}{N}\) досягає максимального значення, і за допомогою обчислення ми можемо визначити, що воно досягає максимального значення\( \frac{15\sqrt{10}}{128}\ =\ 0.3706\) коли\( \theta\ =\ \theta^{-1}\left(\frac{9}{11}\right)=35^{\circ}06'\). Якщо\( \mu\) < 0,3706, нижня частина стрижня буде ковзати раніше\( \theta=35^{\circ}06'\). Якщо, однак,\( \mu\) > 0.3706, стрижень не буде прослизнути на той час\( \theta=35^{\circ}06'\), і це безпечно на деякий час, як\( \frac{F}{N}\) починає зменшуватися. При\( \theta\)\( \cos^{-1}\left(\frac{2}{3}\right)=48^{\circ}11'\) досягненні сила тертя змінює знак і після цього діє вліво. (Зліва діє сила тертя столу на стрижень; справа діє сила тертя штока на столі.) Ми знаємо до теперішнього часу (так як стрижень вижив прослизання до\( \theta=35^{\circ}06'\) цього, величина\( \frac{F}{N}\) може бути принаймні такою ж великою.
як 0.3706, і він не досягає цього поки\( \theta=51^{\circ}15'\). Тому, якщо стрижень hasn 't зісковзнув по\( \theta=51^{\circ}15'\) ньому не буде ковзати раніше\( \theta=51^{\circ}15'\). Але після цього йому загрожує знову зісковзнутися. \( \frac{F}{N}\)стає нескінченним (\( N=0\)) коли\( \theta=\cos^{-1}\left(\frac{1}{3}\right)=70^{\circ}32'\), тому він неодмінно прослизне (праворуч) до цього.
Якщо\( \mu=0.25\), стрижень буде ковзати вліво, коли
\( \frac{3\sin\theta(3\cos\theta-2)}{(1-3\cos\theta)^{2}}\ =\ \frac{1}{4},\ \text{or}\ \underline{\underline{\theta=19^{\circ}39'}}\).
Якщо\( \mu = 0.75\), стрижень буде ковзати вправо, коли
\( \frac{3\sin\theta(3\cos\theta-2)}{(1-3\cos\theta)^{2}}\ =\ -\frac{3}{4},\ \text{or}\ \underline{\underline{\theta=53^{\circ}07'}}\).
Знову ж таки, дуже необхідно, щоб ви підготували для себе програму, яка миттєво вирішить рівняння\( f(x)=0\).
Нехай довжина сходів буде\( 2l\). За геометрією відстань OC залишається рівною\( l\) всьому руху; тому C описує коло радіуса\( l\), центр О. I позначив зеленим кольором радіальну і поперечну складові прискорення С, а саме\( l\dot{\theta}^{2}\) і\( l\ddot{\theta}\). Кутова швидкість сходів є\( \dot{\theta}\) і лінійна швидкість центру мас С дорівнює\( l\ddot{\theta}\). Я також позначив червоним кольором три сили, що діють на сходи, а саме її вага і реакції підлоги і стіни на сходи.центр О. я позначив зеленим кольором радіальну і поперечну складові прискорення С, а саме\( l\dot{\theta}^{2}\) і\( l\ddot{\theta}\). Кутова швидкість сходів є\( \dot{\theta}\) і лінійна швидкість центру мас С дорівнює\( l\ddot{\theta}\). Я також позначив червоним кольором три сили, що діють на сходи, а саме її вагу та реакції підлоги та стіни на сходах.
Кутову швидкість\( \dot{\theta}\) можна отримати з енергетичних міркувань. Тобто втрата потенційної енергії при переході від кута а до вертикалі до кута\( \theta\) дорівнює коефіцієнту посилення поступальної і обертальної кінетичних енергій:
\( m\text{g}l(\cos\alpha-\cos\theta)=\frac{1}{2}m(l\dot{\theta})^{2}\ +\ \frac{1}{2}\left(\frac{1}{2}ml^{2}\right)\dot{\theta}^{2}\).
\[ \dot{\theta}^{2}=\frac{3\text{g}}{2l}(\cos\alpha-\cos\theta). \tag{1}\label{8.1} \]
Кутове прискорення\( \ddot{\theta}\) можна отримати з наступного рівняння:
\[ m\text{g}l\sin\theta=\frac{4}{3}ml^{2}\ddot{\theta} \tag{2}\label{8.2} \]
Виведення рівняння (\( \ref{2}\)) викликає деякі цікаві моменти, і я обговорюю це в Додатку в кінці задачі.
Вертикальні і горизонтальні рівняння руху бувають:
\[ N_{2}\ =\ m(l\ddot{\theta}\cos\theta-l\dot{\theta}^{2}\sin\theta) \tag{3}\label{8.3} \]
і
\[ m\text{g}\ -\ N_{1}\ =m(l\ddot{\theta}\sin\theta\ +\ l\dot{\theta}\cos\theta), \tag{4}\label{8.4} \]
хоча нам потрібні лише перші з них, тому що ми хочемо з'ясувати, коли\( N_{2}\ =\ 0\).
На заміну для\( \ddot{\theta}\) і\( \dot{\theta}^{2}\) ми знаходимо, що
\[ N_{2}\ =\ \frac{3}{4}m\text{g}\sin\theta(3\cos\theta-2\cos\alpha) \tag{5}\label{8.5} \]
і
\[ N_{1}\ =\ \frac{1}{4}m\text{g}(1-6\cos\alpha\cos\theta+9\cos^{2}\theta) \tag{6}\label{8.6} \]
Нам потрібно лише перший з них, щоб побачити, що\( N_{2}\) стає нулем (а значить, верхній кінець втрачає контакт зі стіною), коли\( \cos\theta=\frac{2}{3}\cos\alpha\).
Додаток: Виведення рівняння (2).
У моєму первісному повідомленні цього рішення я отримав Equation (\( \ref{2}\)), вважаючи, що загальний момент всіх сил приблизно\( Q\) є\( m\text{g}l\sin\theta\), і обертальна інерція щодо\( Q\) є\( \frac{4}{3}ml^{2}\). Я тоді\( m\text{g}l\sin\theta\) прирівняв до\( \frac{4}{3}ml^{2}\ddot{\theta}\). Я зобов'язаний кореспонденту Аміну Резаі Заде за те, що він вказував на недолік у цьому аргументі, і за надання правильного виведення. Недолік полягає в тому, що я застосовую рівняння\( \bf{\tau=\dot{L}}\) до рухомої точки\( Q\). У розділі 3.12 глави 3 цих приміток зазначено, що\( \bf{\tau=\dot{L}}\) може бути застосовано до рухомої точки лише в тому випадку, якщо рухома точка задовольняє одній або більше з трьох умов, і в цій задачі очевидно, що жодна з цих умов не\( Q\) задовольняє. Я представляю правильне виведення пана Резаі рівняння (\( \ref{7.2}\)) нижче.
Я буду використовувати рівняння\( \ref{3.12.1}\) і\( \ref{3.12.2}\):
\[ \dot{L}_{Q}\ =\ \tau_{Q}\ +\ Mr'_{q}\ \times\ \ddot{r}_{Q}. \tag{3.12.1}\label{3.12.1} \]
\[ L_{Q}\ =\ \Sigma(r_{i}-r_{Q})\times[m_{i}(v_{i}-v_{Q})]. \tag{3.12.2}\label{3.12.2} \]
Я також використовуватиму позначення, що використовуються в розділі 3.12, і я відтворюю тут малюнок III.7 з цього розділу, і я також намалюю відповідні вектори, відповідні цій проблемі сходів.
На малюнку нижче я вказав елементарну\( ds\) частину сходів на відстані\( s\) від верхнього торця сходів. Маса його, очевидно\( dm=\frac{mds}{2l}\). Я намалював вектори положення\( \bf{r}_{i}\) і\( \bf{r}_{O}\) з\( ds\) і з\( Q\). Це позначення відповідає тим же позначенням, що використовується в Розділі 3.12. З геометрії фігури можна визначити, що
\[ \textbf{r}_{i}\ =\ s\sin\theta\textbf{i}\ +\ (2l-s)\cos\theta\textbf{j} \tag{A1}\label{A1} \]
і
\[ \textbf{r}_{Q}\ =\ 2l\sin\theta\textbf{i}\ +\ 2l\cos\theta\textbf{j}, \tag{A2}\label{A2} \]
де\( \textbf{i}\) і\( \textbf{j}\) є одиничними векторами в\( x-\) і\( y-\) напрямках відповідно.
Про диференціювання по відношенню до часу знаходимо наступні вирази для швидкостей елемента\( ds\) і точки\( Q\), в яких я знову зберігаю позначення, використані в розділі 3.12:
\[ \textbf{v}_{i}\ =\ s\dot{\theta}\cos\theta\textbf{i}\ -\ (2l-s)\dot{\theta}\sin\theta\textbf{j} \label{A3}\tag{A3} \]
і
\[ \textbf{v}_{Q}\ =\ 2l\dot{\theta}\cos\theta\textbf{i}\ -\ 2l\dot{\theta}\sin\theta\textbf{j} \label{A4}\tag{A4} \]
Використовуючи рівняння\( \ref{3.12.2}\), отримаємо для кутового моменту елемента\( ds\) щодо\( Q\):
\[ d\textbf{L}_{Q}\ =\ \frac{m}{2l}(\textbf{r}_{i}-\textbf{r}_{Q})\times[(\textbf{v}_{i}-\textbf{v}_{Q})]ds. \tag{A5}\label{A5} \]
Таким чином, миттєвий кутовий імпульс всієї сходи\( Q\) приблизно
\[ \textbf{L}_{Q}\ =\ \frac{m}{2l}\int_{0}^{2l}(\textbf{r}_{i}-\textbf{r}_{Q})\times[(\textbf{v}_{i}-\textbf{v}_{Q})]ds. \tag{A6}\label{A6} \]
При заміщенні рівнянь (\( \ref{A1}\)) — (\( \ref{A4}\)) на рівняння (\( \ref{A6}\)) та скромну величину алгебри отримано
\[ \textbf{L}_{Q}\ =\ \frac{m\theta\textbf{k}}{2l}\int_{0}^{2l}s(s-2l)ds\ =\ -\frac{2}{3}ml^{2}\dot{\theta}\textbf{k}, \label{A7}\tag{A7} \]
де\( \textbf{k}\) - одиничний вектор в\( z-\) напрямку. (\( z-\)Напрямок виходить з площини «паперу», а тому\( \bf{L}_{Q}\) знаходиться в площині «паперу». Варто витратити хвилинку або дві спроби собі це уявити. Сходи обертаються проти годинникової стрілки близько C, в той час як C і Q рухаються за годинниковою стрілкою траєкторій. Це може бути не відразу очевидним вирішити, чи слід очікувати,\( \bf{L}_{Q}\) щоб бути спрямованим в площину «паперу» або з неї. Рівняння (\( \ref{A7}\)) відповідає на це питання.)
Тепер ми використовуємо рівняння\( \ref{3.12.1}\):
\[ \dot{\bf{L}}_{Q}\ =\ \tau_{Q}\ +\ m\bf{r'}_{Q}\times\bf{\ddot{r}}_{Q}. \tag{A8}\label{A8} \]
Знайдемо вирази для чотирьох векторних величин у цьому рівнянні.
Шляхом диференціації рівняння (\( \ref{A7}\)) щодо часу отримано
\[ \dot{\bf{L}}_{Q}\ =\ -\frac{2}{3}ml^{2}\ddot{\theta}\textbf{k}. \tag{A9}\label{A9} \]
Крутний момент близько Q дорівнює
\[ \tau_{Q}\ =\ mgl\sin\theta\textbf{k} \tag{A10}\label{A10} \]
Ми можемо бачити з геометрії фігури (див. Особливо другу нашу фігуру, в якій ми бачимо, що\( \textbf{r}'_{Q}\) і\( \overline{\textbf{r}}\) однакові за величиною та напрямком), що
\[ \textbf{r}^{\prime}_{Q}\ =\ l\sin\theta\textbf{i}\ +\ l\cos\theta\textbf{j}. \tag{A11}\label{A11} \]
Нарешті, шляхом диференціації рівняння (\( \ref{A4}\)) (в якому\( \)) отримаємо
\[ \ddot{\textbf{r}}_{Q}\ =\ 2l[(\ddot{\theta}\cos\theta-\dot{\theta}^{2}\sin\theta)\textbf{i}\ -\ (\ddot{\theta}\sin\theta-\dot{\theta}^{2}\cos\theta)\textbf{j}]. \tag{A12}\label{A12} \]
Заміна рівнянь (\( \ref{A9}\)) до (\( \ref{A12}\)) на рівняння (\( \ref{A8}\)) дає після деякої алгебри,
\[ mgl\sin\theta\ =\ \frac{4}{3}ml^{2}\ddot{\theta}. \tag{A13}\label{A13} \]
Це Рівняння (\( \ref{2}\)), який є демонстрантом.
Я думаю, буде домовлено, що точка О залишається нерухомою в просторі до тих пір, поки півциліндр залишається в контакті зі стіною і підлогою. Тому центр маси C рухається по колу навколо О. Ми будемо називати радіус кола, який є відстанню між O і C\( b\), що для півциліндра дорівнює центру маси C рухається по колу навколо О. Ми будемо називати радіус кола, який є відстанню між O і C, \( b\), Який для напівциліндра дорівнює\( \frac{4a}{(3\pi)}\) (див. Главу 1), де\( a\) - радіус півциліндра. Я позначив червоним кольором три сили на півциліндрі, а також, зеленим кольором, радіальну і поперечну складові прискорення.
Кутову швидкість\( \dot{\theta}\) можна отримати з енергетичних міркувань. Приріст кінетичної енергії при переході від спокою до кутової швидкості\( \dot{\theta}\) є\( \frac{1}{2}\left(mk^{2}\right)\dot{\theta}^{2}\) і посилення потенційної енергії, коли центр маси падає через вертикальну відстань\( b\ \sin\theta\)\( mgb\sin\theta\). \( k\)Ось радіус обертання близько О, який для півциліндра задається\(k^2 = \frac{1}{2}a^2\).
[Я пішов\( b\) and \( k\) as they are in the equations, so that the analysis could easily be adapted, if needed, for a hollow semicylinder, or a solid hemisphere, or a hollow hemisphere. From Chapters 1 and 2 we recall:
| Суцільний напівциліндр: | \( b=\frac{4a}{3\pi}\) | \( k^{2}\ =\ \frac{1}{2}a\) | \( \frac{b^{2}}{k^{2}}\ =\ \frac{32}{9\pi^{2}}\) |
| Порожній напівциліндр: | \( b=\frac{2a}{\pi}\) | \( k^{2}\ =\ a^{2}\) | \( \frac{b^{2}}{k^{2}}\ =\ \frac{4}{\pi^{2}}\) |
| Тверда півсфера: | \( b=\frac{3a}{8}\) | \( k^{2}\ =\ \frac{2}{5}a^{2}\) | \( \frac{b^{2}}{k^{2}}\ =\ \frac{45}{128}\) |
| Порожниста півсфера: | \( b=\frac{1}{2}a\) |
\( k^{2}\ =\ \frac{2}{3}a^{2}\) |
\( \frac{b^{2}}{k^{2}}\ =\ \frac{3}{8}\) |
Про прирівнювання посилення кінетичної енергії до втрати в потенційній енергії отримаємо
\[ \dot{\theta}^{2}\ =\ \frac{2b\text{g}}{k^{2}}\sin\theta. \tag{1}\label{9.1} \]
Кутове прискорення\( \ddot{\theta}\) можна отримати при застосуванні\( \tau\ =\ I\ddot{\theta}\) близько O:
\( m\text{g}b\cos\theta\ =\ mk^{2}\ddot{\theta}\),
з якого
\[ \ddot{\theta}\ =\ \frac{bg}{k^{2}}\cos\theta \tag{2}\label{9.2} \]
Горизонтальні і вертикальні рівняння руху
\[ N_{2}\ =\ mb(\dot{\theta}^{2}\cos\theta\ + \ddot{\theta}\sin\theta) \tag{3}\label{9.3} \]
і
\[ N_{2}\ =\ mb(\dot{\theta}^{2}\cos\theta\ + \ddot{\theta}\sin\theta) \tag{4}\label{9.4} \]
Нам насправді не потрібно Equation (\( \ref{4}\)), тому що ми намагаємося визначити, коли\( N_{2}=0\).
При заміщенні з рівнянь (\( \ref{1}\)) та (\( \ref{2}\)) рівняння (\( \ref{3}\)) стає
\[ N_{1}=\frac{6mb^{2}\text{g}}{a^{2}}\sin\theta\cos\theta. \tag{5}\label{9.5} \]
Це нуль коли\( \theta\ =\ 0^{\circ}\) (яке було початковою умовою) або коли\( \theta\ =\ 0^{\circ}\), в якій точці втрачається контакт зі стіною, який потрібно було показати.
У цей момент швидкість обертання йде\( \sqrt{\frac{2b\text{g}}{k^{2}}}\) проти годинникової стрілки.
а лінійна швидкість С\( b\sqrt{\frac{2b\text{g}}{k^{2}}}\) горизонтально вправо.
Обертальна кінетична енергія\( I\) є\( \frac{1}{2}I\omega^{2}\) де\( \omega\ =\ \sqrt{\frac{2b\text{g}}{k^{2}}}\), і є обертальною інерцією навколо центру маси, який є\( m(k^{2}-b^{2})\).
\( K_{\text{rot}}=\frac{mb\text{g}(k^{2}-b^{2})}{k^{2}}\).
Поступальна кінетична енергія - це\( \frac{1}{2}mv^{2}\) де\( v\ =\ \sqrt{\frac{2bg}{k^{2}}}\).
\( K_{\text{tr}}\ =\ \frac{mb^{3}\text{g}}{k^{2}}\)
Сума їх дорівнює\( mb\text{g}\), яка якраз дорівнює втраті вихідної потенційної енергії, яка служить перевіркою на правильність нашої алгебри.
Горизонтальних сил зараз немає, тому горизонтальна складова швидкості С залишається постійною. Однак напівциліндр продовжує обертатися до тих пір, поки обертальна кінетична енергія не перетвориться в потенційну енергію і С не підніметься на максимальну висоту. Якщо основа потім робить кут\( \phi\) з вертикаллю, посилення потенційної енергії є\( mb\text{g}\sin\phi\), і прирівнюючи це до обертальної кінетичної енергії дає
\( \sin\phi\ =\ 1\ -\ \frac{b^{2}}{k^{2}}\).
Це дає наступні результати:
| Суцільний напівциліндр: | \( \phi\ =\ 39^{\circ}\ 46'\) |
| Порожній напівциліндр: | \( \phi\ =\ 36^{\circ}\ 30'\) |
| Тверда півсфера: | \( \phi\ =\ 40^{\circ}\ 25'\) |
| Порожниста півсфера: | \( \phi\ =\ 38^{\circ}\ 41'\) |
Додайте сюди текст. Щоб автоматичний номер працював, потрібно додати шаблон «AutoNum» (бажано в кінці) на сторінку.
Загальновідомо, що якщо\( \alpha\ >\ \tan^{-1}\mu\) частинка буде ковзати вниз по площині, якщо не допоможе додаткова сила. Я намалював три сили, що діють на частинку. Його вага\( mg\). \( R\)Реакція площини на частинку; якщо частка знаходиться в граничній статичній рівновазі, ця реакція зробить кут\( \lambda\) («кут тертя») з площиною такий, що\( \tan\lambda\ =\ \mu\). Тому він робить кут\( \alpha-\theta\) з вертикаллю. Нарешті,\( P\) потрібна додаткова сила; ми спочатку не знаємо напрямок цієї сили.
Коли три (або більше) компланарні сили знаходяться в рівновазі і намальовані головою до хвоста, вони утворюють замкнутий трикутник (багатокутник). Трикутник сил малюю нижче.
З трикутника буде зрозуміло, що\( P\) is least when the angle between \( \textbf{P}\) and \( \textbf{R}\) is \( 90^{\circ}\):
The least value of \( P\) is therefore \( m\text{g}(\sin\alpha\cos\lambda\ -\ \cos\alpha\sin\lambda)\). But \( \tan\lambda=\mu\) and therefore \( \sin\lambda=\frac{\mu}{\sqrt{1+\mu^{2}}}\) and \( \cos\lambda=\frac{1}{\sqrt{1+\mu^{2}}}\).
\( P_{\text{min}}=\frac{m\text{g}(\sin\alpha-\mu\cos\alpha)}{\sqrt{1-\mu^{2}}}\)
and \( P\) then makes an angle \( \lambda\) with the plane.
You may, if you wish, go further, and show that when \( \textbf{P}\) makes an angle \( \beta\) with the plane, it must have magnitude
\( P=\frac{\sin\alpha-\mu\cos\alpha}{\mu\sin\beta\ +\ \cos\beta}m\text{g}\).
You can then differentiate this with respect to \( \beta\) (you need only differentiate the denominator) and show that this is a minimum when \( \beta\ =\ \lambda\). That is just a harder way of finding what we already found by using the triangle of forces.
For \( \alpha\ =\ 70^{\circ}\) and \( \mu\ =\ 0.8\), \( P\) varies with \( \beta\) like this:
This goes through a minimum of \( P_{\text{min}}=0.520m\text{g}\) at \( \beta\ =\ \tan^{-1}0.8=38^{\circ}.7\).
_________________________
Add text here. For the automatic number to work, you need to add the "AutoNum" template (preferably at the end) to the page.
As the cylinder rolls down the plane, the wedge, because its base is smooth, will slide towards the left. Since there are no external horizontal forces on the system, the centre of mass of the system will not move horizontally (or, rather, it won’t accelerate horizontally.)centre of mass of the system will not move horizontally (or, rather, it won’t accelerate horizontally.)
As usual, we draw a large diagram, using a ruler , and we mark in the forces in red and the accelerations in green, after which we’ll apply \( F\ =\ ma\) to the cylinder, or to the wedge, or to the system as a whole, in two directions. It should be easy and straightforward.
I have drawn the linear acceleration \( \ddot{s}\) of the cylinder down the slope, and its angular acceleration \( \ddot{\theta}\). I have drawn the linear acceleration \( \ddot{x}\) of the wedge, which is also shared with the cylinder. I have drawn the gravitational force \( mg\) on the cylinder. There is one more force on the cylinder, namely the reaction of the wedge on the cylinder. But I’m not sure in which direction to draw it. Is it normal to the plane? That would mean there is no frictional force between the cylinder and the plane. Is that correct (remembering that both the cylinder and the wedge are accelerating)? Of course I could calculate the moment of the force \( mg\) about the point of contact of the cylinder with the plane, and then I wouldn’t need to concern myself with any forces at that point of contact.
But then that point of contact is not fixed. Oh, dear, I’m getting rather muddled and unsure of myself.
This problem, in fact, is ideally suited to a lagrangian rather than a newtonian treatment, and that is what we shall do. Lagrange proudly asserted that it was not necessary to draw any diagrams in mechanics, because it could all be done analytically. We are not quite so talented as Lagrange, however, so we still need a large diagram drawn with a ruler. But, instead of marking in the forces and accelerations in red and green, we mark in the velocities in blue.
No frictional or other nonconservative forces do any work, so we can use Lagrange’s equations of motion for a conservative holonomic system; \( \frac{d}{dt}\left(\frac{\partial T}{\partial \dot{q}}\right)-\left(\frac{\partial T}{\partial q}\right)=-\left(\frac{\partial V}{\partial q}\right)\).
The speed of the wedge is \( \dot{x}\) and the speed of the centre of mass of the cylinder is centre of mass of the cylinder is \( \sqrt{\dot{s}^{2}+\dot{x}^{2}-2\dot{s}\dot{x}\cos\alpha}\) and the angular speed of the cylinder is \( \frac{\dot{s}}{a}\).
The kinetic energy of the system is
\( T\ =\ \frac{1}{2}m(\dot{s}^{2}+\dot{x}^{2}-2\dot{s}\dot{x}\cos\alpha)\ +\ \frac{1}{2}(mk^{2})\left(\frac{\dot{s}}{a}\right)\ +\ \frac{1}{2}M\dot{x}^{2}\),
or
\( T\ =\ \frac{1}{2}m\left(1+\frac{k^{2}}{a^{2}}\right)\dot{s}^{2}\ -\ m\dot{s}\dot{x}\cos\alpha\ +\ \frac{1}{2}(m\ +\ M)\dot{x}^{2}\),
and the potential energy is
\( V\ =\ \text{constant}\ -\ m\text{g}s\sin\alpha\)
Application of Lagrange’s equation to the coordinate \( x\) gives us
\( \left(1+\frac{k^{2}}{a^{2}}\right)\ddot{s}=\ddot{x}\cos\alpha\ +\ \text{g}\sin\alpha\).
and application of Lagrange’s equation to the coordinate \( s\) gives us
\( m\ddot{s}\cos\alpha\ =\ (m+M)\ddot{x}\)
Elimination of \( \ddot{s}\) from these two equations gives us
\( \ddot{x}=\frac{m\text{g}\sin\alpha\cos\alpha}{(m+M)\left(1+\frac{k^{2}}{a^{2}}\right)-m\cos^{2}\alpha}\)
You can also easily find an expression for \( \ddot{s}\) is you wish.
There is no acceleration normal to the plane, and therefore \( N\ =\ m\text{g}\cos\alpha\). The frictional force \( F\) acts along the tangent to the path and is equal to \( \mu N\), or \( \mu m\text{g}\cos\alpha\), where \( \mu\) is the coefficient of moving friction. We are told to ignore the difference between the coefficients of moving and limiting static friction. Since the particle was originally at rest in limiting static friction, we must have \( \mu\ =\ \tan\alpha\). Therefore \( F\ =\ m\text{g}\sin\alpha\). The tangential equation of motion is
\( m\ddot{s}\ =\ -F\ +\) whatever the component of \( m\textbf{g}\) is in the tangential direction in the sloping plane.
The component of \( m\textbf{g}\) down the plane would be (look at the left hand drawing) \( m\text{g}\sin\alpha\), and so its tangential component (look at the right hand drawing) is \( m\text{g}\sin\alpha\sin\psi\). So we have, for the tangential equation of motion,
\( m\ddot{s}=-m\text{g}\sin\alpha\ +\ m\text{g}\sin\alpha\sin\psi\),
or
\( \ddot{s}=-\text{g}\sin\alpha(1-\sin\psi)\).
We are seeking a relation between \( V\) and \( \psi\), so, in the now familiar fashion, we write \( V\frac{dV}{ds}\) for \( \ddot{s}\), so the tangential equation of motion is
\[ V\frac{dV}{ds}=-\text{g}\sin\alpha(1-\sin\psi). \tag{1}\label{12.1} \]
We also need the equation of motion normal to the trajectory. The component of \( m\textbf{g}\) in that direction is \( m\text{g}\sin\alpha\cos\psi\), and so the normal equation of motion is
\( \frac{mV^{2}}{\rho}\ =\ m\text{g}\sin\alpha\cos\psi\).
Here \( \rho\) is the radius of curvature of the path, which is the reciprocal of the curvature \( \frac{ds}{d\psi}\). The normal equation of motion is therefore
\[ V^{2}\frac{d\psi}{ds}\ =\ \text{g}\sin\alpha\cos\psi. \tag{2}\label{12.2} \]
Divide Equation (\( \ref{1}\)) by Equation (\( \ref{2}\)) to eliminate \( s\) and thus get a desired differential equation between \( V\) and \( \psi\):
\[ \frac{1}{V}\frac{dV}{d\psi}\ =\ -\frac{(1-\sin\psi)}{\cos\psi}. \tag{3}\label{12.3} \]
This is easily integrated; a convenient (not the only) way is to multiply top and bottom by \( 1\ +\ \sin\psi\). In any case we soon arrive at
\[ \ln V\ =\ -\ln(1+\sin\psi)\ +\ \text{constant}, \tag{4}\label{12.4} \]
and with the initial condition \( V\ =\ V_{0}\) when \( \psi\ =\ 0\), this becomes
\[ \underline{\underline{V=\frac{V_{0}}{1+\sin\psi}}}. \tag{5}\label{12.5} \]
In the limit, as \( \psi\rightarrow90^{\circ},\ V\rightarrow\frac{1}{2}V_{0}\). The particle is then moving at constant velocity and is in equilibrium under the forces acting upon it just when it was initially at rest.
13.
\( M_{1}\) = mass of complete sphere of radius \( a\).
\( M_{1}\) = mass of missing inner sphere of radius \( xa\).
\( M\) = mass of given hollow sphere.
We have \( M\ =\ M_{2}-M_{1}\) and \( \frac{M_{2}}{M_{1}}=x^{3}\) and therefore
\( M_{1}\ =\ \frac{M}{1-x^{3}}\) and \( M_{2}\ =\ \frac{Mx^{3}}{1-x^{3}}\).
Also \( I=\frac{2}{5}M_{1}a^{2}\ -\ \frac{2}{5}M_{2}x^{2}a^{2}\ =\ \frac{2}{5}a^{2}(M_{1}-M_{2}x^{2})\).
Hence \( I=\frac{2}{5}Ma^{2}\times\frac{1-x^{5}}{1-x^{3}}\).
If \( x=0,\ I\ =\ \frac{2}{5}Ma^{2}\), as expected. If \( x\rightarrow1\), you may have to use de l’Hôpital’s rule to show that \( I\rightarrow\frac{2}{5}Ma^ {2}\) as expected.
\( M_{1}\) = mass of mantle.
\( M_{2}\) = mass of core.
\( M\) = mass of entire planet.
We have \( M=M_{1}+M_{2}\) and \( \frac{M_{1}}{M_{2}}=\frac{s(1-x^{3})}{x^{3}}\) and therefore
\( M_{2}\ =\ M\times\frac{x^{3}}{x^{3}\ +\ s(1-x^{3})}\) and \( M_{1}\ =\ M\times\frac{s(1-x^{3})}{x^{3}\ +\ s(1-x^{3})}\)
Also
\( I\ =\ I_{\text{core}}\ +\ I_{\text{mantle}}\ =\ \frac{2}{5}M_{2}x^{2}a^{2}\ +\ \frac{2}{5}M_{1}a^{2}\times\frac{1-x^{5}}{1-x^{3}}\),
where I have made use of the result from the previous problem. On substitution of the expressions for \( M_{1}\) and \( M_{2}\), we quickly obtain
\[ I\ =\ \frac{2}{5}Ma^{2}\times\frac{s\ +\ (1-s)x^{5}}{s\ +\ (1-s)x^{3}} \tag{1}\label{14.1} \]
A hollow planet would correspond to \( \frac{1}{s}=0\). Divide top and bottom by \( s\) and it is immediately seen that the expression for a hollow planet would be identical to the expression obtained for the previous problem.
Note that both \( x\ =\ 0\) and \( x\ =\ 1\) correspond to a uniform sphere, so that in either case, \( I\ =\ \frac{2}{5}Ma^{2}\) for all other cases, the moment of inertia is less than \( \frac{2}{5}Ma^{2}\).
The core size for minimum moment of inertia is easily found by differentiation of the above expression for \( I\), and the required expression follows after some algebra. For \( s\ =\ 0.6\), the equation becomes \( 9-15x^{2}-4x^{5}\ =\ 0\), of which the only positive real root is \( x\ =\ 0.73682\), which corresponds to a moment of inertia of 0.90376 % \( \frac{2}{5}Ma^{2}\). Note that. for \( s\ =\ 0.6\), the moment of inertia, expressed in units of \( \frac{2}{5}Ma^{2}\) varies very little as the core size goes from 0 to 1, so that measurement of the moment of inertia places very little restriction on the possible core size.
The inverse of Equation (\( \ref{1}\)) is
\[ (1-s)x^{5}\ -\ I(1-s)x^{3}\ +\ (1-I)s\ =\ 0, \tag{2}\label{14.2} \]
where \( I\) is expressed in units of \( \frac{2}{5}Ma^{2}\). For \( I\ =\ 0.911\), there are two positive real roots (look at the graph); they are \( x\ =\ 0.64753\) and \( 0.81523\). For \( I\ =\ 0.929\), the roots are 0.55589 and 0.87863. Thus the core size could be anything between 0.55589 and 0.64753 or between 0.81523 and 0.87863 a rather large range of uncertainty. Even if \( I\) were known exactly (which does not happen in science), there would be two solutions for \( x\).
This is just a matter of geometry. If, when you make a small angular displacement, you raise the centre of mass of the brick the equilibrium is stable. For, while the brick is in its vertical position, it is evidently at a potential minimum, and you have to do work to raise the centre of mass. If, on the other hand, your action in making a small angular displacement results in a lowering of the centre of mass, the equilibrium is unstable.centre of mass of the brick the equilibrium is stable. For, while the brick is in its vertical position, it is evidently at a potential minimum, and you have to do work to raise the centre of mass. If, on the other hand, your action in making a small angular displacement results in a lowering of the centre of mass, the equilibrium is unstable.
When the brick is in its vertical position, the height \( h_{0}\) of its centre of mass above the base of the semicylinder is justcentre of mass above the base of the semicylinder is just
\( h_{0}\ =\ R\ +\ l\).
When it is displaced from the vertical by an angle \( \theta\), the point of contact between brick and semicylinder is displaced by a distance \( R\theta\), and, by inspection of the drawing, the new height \( h\) is
\( h\ =\ R\cos\theta\ +\ R\theta\sin\theta\ +l\cos\theta\).
\( h-h_{0}\ =\ R\theta\sin\theta\ -\ (R+l)(1-\cos\theta)\).
If you Maclaurin expand this as far as \( \theta^{2}\), you arrive at
\( h-h_{0}\approx\frac{1}{2}(R-l)\theta^{2}\).
This is positive, and therefore the equilibrium is stable, if \( l\ <\ R\), or \( 2l\ <\ 2R\), i.e. if the length of the brick is less than the diameter of the semicylinder.
As in the previous question, it is just a matter of geometry. If rolling the Thing results in raising its centre of mass, the equilibrium is stable. Initially, the height of the centre of mass is \( H_{0}\ =\ b\ + l\).centre of mass, the equilibrium is stable. Initially, the height of the centre of mass is \( H_{0}\ =\ b\ + l\).
After rolling, the dashed line, which joins the centres and is of length \( a+b\), makes an angle \( \theta\) with the vertical. The short line joining the centre of mass of the Thing to the centre of curvature of its bottom is of length \( l-a\) and it makes an angle \( \theta+\\phi\) with the vertical. The height of the centre of mass is therefore nowcentre of mass of the Thing to the centre of curvature of its bottom is of length \( l-a\) and it makes an angle \( \theta+\\phi\) with the vertical. The height of the centre of mass is therefore now
\( h\ =\ (a+b)\cos\theta+(l-a)\cos(\theta+\phi)\)
The centre of mass has therefore rise through a heightcentre of mass has therefore rise through a height
\( h\ -\ h_{0}\ = (a+b)\cos\theta\ +\ (l-a)\cos(\theta+\phi)\ -b-l\).
Also, the two angles are related by \( a\phi\ =\ b\theta\), so that
\( h\ -\ h_{0}\ = (a+b)\cos\theta\ +\ (l-a)\cos\left[\left\{1+\left(\frac{b}{a}\right)\right\}\theta\right]\ -b-l\)
\( h\ -\ h_{0}\ = -\frac{1}{2}\theta^{2}[a+b\ +\ (l-a)(1+\frac{b}{a})^{2}]\).
For stability this must be positive, and hence \( \frac{1}{l}\ >\ \frac{1}{a}\ +\ \frac{1}{b}\).
If \( a\ =\ b\), this becomes \( l\ <\ \frac{1}{2}a\).
| For a hollow semicylinder, | \( l\ =\ (1-\frac{2}{\pi})a=0.363a\) | \( \Box\) Stable |
| For a hollow hemisphere, | \( l=0.5a\) | \( \Box\) Borderline stable |
| For a solid semicylinder, | \( l\ =\ [1-\frac{4}{(3\pi)}]a=0.576a\) | \( \Box\) Unstable |
| For a solid hemisphere, | \( l=\frac{5}{8}a=0.625a\) | \( \Box\) Unstable |
We need to find the height \( h\) of the centre of mass above the level of the pegs as a function of \( \theta\). See drawing on next page.centre of mass above the level of the pegs as a function of \( \theta\). See drawing on next page.
Angels:
\( \text{BAC}\ =\ 45^{\circ}-\theta\)
\( \text{ABX}\ =\ 45^{\circ}+\theta\)
Distances:
\( \text{AB}\ =\ 2ka\)
\( \text{AC}\ =\ 2ka\ \cos(45^{\circ}-\theta)\)
\( \text{EF}\ =\ 2ka\ \cos(45^{\circ} -\theta)\cos(45^{\circ}+\theta)=ak\cos2\theta\)
\( \text{DC}\ =\ a\sqrt{2}\)
\( \text{DF}\ =\ a\sqrt{2}\cos\theta\)
\( h\ =\ \text{DF}\ -\ \text{EF}\ =\ a(\sqrt{2}\cos\theta-k\cos2\theta)\)
\( h_{0}=\) height of centre of mass above pegs when \( \theta = 0 \) centre of mass above pegs when \( \theta\ =\ 0^{\circ}\ =\ a(\sqrt{2}-k)\)
\( y\ =\ \frac{h}{h_{0}}\ =\frac{\sqrt{2}\cos\theta-k\cos2\theta}{\sqrt{2}-k}\)
There are three forces acting on the hemisphere: Its weight \( m\text{g}\). The reaction \( N\) of the wall, which is perpendicular to the wall since the wall is smooth. The reaction \( R\) of the floor, which acts at an angle \( \lambda\) to the floor, where \( \mu\ =\ \tan\lambda\). Three forces in equilibrium must act through a point; therefore all three forces act through the point \( P\). It is thus clear that
\( \sin\ \theta\ =\ \frac{\text{OP}}{\text{OC}}\ =\ \frac{a\mu}{\frac{3}{8}a}\ =\ \frac{8\mu}{3}\).
If \( \mu\ =\ \frac{1}{4},\ \theta\ =\ 41^{\circ}48'\). If \( \mu\ =\ \frac{3}{8},\ \theta\ =\ 90^{\circ}\). If \( \mu\ >\ \frac{3}{8}\) the hemisphere can rest in any position, the equilibrium not being limiting static equilibrium.
This solution uses the same method that Professor Marsh (Warwick University) showed me for Problem 20. I believe it to be clearer than an earlier solution that I had posted.
At an instant when the rod is tilted at angle \( \theta\), the coordinates of C with respect to the fixed point O are:
\[ \overline{x}\ =\ a(\sin\theta\ -\ \theta\cos\theta), \tag{1}\label{19.1} \]
\[ \overline{y}\ =\ a(\cos\theta\ +\ \theta\sin\theta) \tag{2}\label{19.2} \]
and so its velocity components are
\[ \dot{\overline{x}}\ =\ a\theta\sin\theta\dot{\theta} \tag{3}\label{19.3} \]
and
\[ \dot{\overline{y}}\ =\ a\theta\cos\theta\dot{\theta}. \tag{4}\label{19.4} \]
The moment of inertia of the rod about the centre of mass is \( \frac{1}{3}ml^{2}\).
The kinetic energy \( T\) is the sum of the translational kinetic energy and the rotational kinetic energy about the centre of mass:centre of mass:
\[ T\ =\ \left(\frac{1}{2}a^{2}\theta^{2}\ +\ \frac{1}{6}l^{2}\right)m\dot{\theta}^{2}, \tag{5}\label{19.5} \]
and the potential energy \( V\) is
\[ V\ =\ m\text{g}a(\cos\ \theta\ +\ \theta\sin\ \theta). \tag{6}\label{19.6} \]
One can now get the equation of motion either by Lagrangian means or by equating the derivative with respect to \( \theta\) of the total energy to zero, since there are no nonconservative forces and hence the total energy is independent of \( \theta\). In carrying out the differentiation, note that \( \frac{d}{d\theta}\dot{\theta}^{2}\ =\ 2\dot{\theta}\frac{d\dot{\theta}}{d\theta}\ =\ 2\ddot{\theta}\). We obtain, for the equation of motion:
\[ a^{2}\theta\dot{\theta}^{2}\ +\ (a^{2}\theta^{2}+\frac{2}{3}l^{2})\ddot{\theta}\ +\ \text{g}a\theta\cos\theta\ =\ 0 \tag{7}\label{19.7} \]
For small \( \theta\) (neglecting second and higher powers of \( \theta\)), \( \cos\theta\rightarrow 1\) and \( a^{2}\theta^{2}\) is negligible compared with \( l^{2}\), so the equation of motion becomes, approximately, \( \ddot{\theta}=-\frac{3\text{g}a}{l^{2}}\), and so the period is \( \underline{\underline{P\ =\ \frac{2\pi l}{\sqrt{3\text{g}a}}}}\).
I am much indebted to Professor T. R. Marsh of Warwick University not only for finding a mistake in an earlier posted solution to this problem, but for providing the following solution.
\[ I\ =\ ma^{2}\left(\frac{7}{5}\ -\ \frac{3}{4}\cos\theta\right). \tag{2}\label{20.2b} \]
\( x\ =\ \frac{3a\theta}{8}\)
We are going to refer the motion to a fixed point Q, which is the point of contact between hemisphere and table when the hemisphere is in its equilibrium position.
At an instant when the hemisphere is tilted at an angle \( \theta\), the distance between A and Q is \( a\theta\), and the coordinates of C relative to Q are
\[ \overline{x}\ =\ a\theta\ -\ \frac{3}{8}a\sin\theta, \tag{1}\label{20.1} \]
\[ \overline{y}\ =\ a -\ \frac{3}{8}a\cos\theta. \tag{2}\label{20.2} \]
Therefore the velocity components of C are
\[ \dot{\overline{x}}\ =\ a\left(1-\frac{3}{8}\cos\theta\right)\dot{\theta}, \tag{3}\label{20.3} \]
\[ \dot{\overline{y}}\ =\ \frac{3}{8}a\sin\theta\dot{\theta}. \tag{4}\label{20.4} \]
By the parallel axes theorem, the moment of inertia around the centre of mass iscentre of mass is
\[ I\ =\ \frac{2}{5}ma^{2}\ -\ m\left(\frac{3}{8}a\right)^{2}\ =\ \frac{83}{320}ma^{2} \tag{5}\label{20.5} \]
The kinetic energy \( T\) is the sum of the translational kinetic energy and the rotational kinetic energy about the centre of mass:centre of mass:
\[ T\ =\ \frac{1}{2}ma^{2}\left[\left(1-\frac{3}{8}\cos\theta\right)^{2}\ +\ \left(\frac{3}{8}\sin\theta\right)^{2}\ +\ \frac{83}{230}\right]\dot{\theta}^{2}\ =\ ma^{2}\left(\frac{7}{10}-\frac{3}{8}\cos\theta\right)\dot{\theta}^{2} \tag{6}\label{20.6} \]
The potential energy \( V\) is
\[ V\ =\ m\text{g}a(1-\frac{3}{8}\cos\theta). \tag{7}\label{20.7} \]
We can the get the equation of motion either by using the Lagrangian equations, or by calculating the derivative with respect to \( \theta\) of the total energy \( T\ +\ V\). The derivative is zero, because there are no nonconservative forces and total energy is constant. Note that (as in Problem 19) the derivative of \( \dot{\theta}^{2}\) with respect to \( \theta\) is \( 2\dot{\theta}\frac{d\dot{\theta}}{d\theta}\), which is \( 2\ddot{\theta}\). Either method results in the equation of motion:
\[ \left(\frac{7}{5}\ -\ \frac{3}{4}\cos\theta\right)\ddot{\theta}\ + \frac{3}{8}\sin\theta\dot{\theta}^{2}\ + \frac{3}{8}\text{g}\sin\theta\ = 0. \tag{8}\label{20.8} \]
In the small angle limit, \( \cos\theta\rightarrow 1\), and \( \sin\theta\rightarrow \theta\) is negligible compared with \( \text{g}\), so the equation of motion becomes
\[ \ddot{\theta}\ =\ -\frac{15\text{g}}{26a}\theta \tag{9}\label{20.9} \]
\[ \underline{\underline{P\ =\ 2\pi\sqrt{\frac{26a}{15\text{g}}}}}. \tag{10}\label{20.10} \]
The (second) moment of inertia with respect to the centre (see centre (see Section 2.19 of Chapter 2) is
\( I_{\text{centre}} = 4 \pi \rho_0 \int^a_0 (r^4-r^5/a)dr = \frac{2}{15}\pi\rho_0a^5.\).
The moment of inertia with respect to an axis through the centre is 2/3 of this:centre is 2/3 of this:
\( I_{\text{axis}}\ =\ \frac{4}{45}\pi\rho_{0}a^{5}\).
\( \therefore \qquad \qquad \qquad \underline{\underline{I_{\text{axis}}\ =\ \frac{4}{15}Ma^{2}}}\).
Left-hand particle:\( T\ =\ m\text{g}[\mu\cos(\alpha-\theta)\ +\ \sin(\alpha-\theta)]\).
Right-hand particle:\( T\ =\ m\text{g}[\sin(\alpha+\theta)\ -\ \mu\cos(\alpha+\theta)]\).
\( \therefore \qquad \mu[\cos(\alpha-\theta)\ +\ \cos(\alpha+\theta)]\ =\ \sin(\alpha+\theta)\ -\ \sin(\alpha-\theta)\),
and, by the “sum and difference” trigonometrical formulae, we obtain
\( 2\mu\cos\alpha\cos\theta\ =\ 2\cos\alpha\sin\theta,\)
from which
\( \underline{\underline{\tan\ \theta\ =\ \mu.}}\)
Consider a portion of the rope between \( \theta\) and \( \delta\theta\). There are four forces on this portion. The tension \( T\) at \( \theta\). The tension \( T\ +\ \delta T\) at \( \theta\ +\ \delta\theta\) (\( \delta T\) is negative). The normal reaction \( \delta N\) of the cylinder on the rope. The frictional force \( \mu\delta N\) of the cylinder on the rope. Note that the rope is about to slip downwards, so the friction force is upwards as shown.
We have
\( \delta N\ =\ (2T\ +\ \delta T)\sin\left(\frac{1}{2}\theta\right)\)
and
\( (T\ +\ \delta T)\cos\left(\frac{1}{2}\delta\theta\right)\ +\ \mu\delta N\ =\ T\cos\left(\frac{1}{2}\delta\theta\right)\).
To first order, these become
\( \delta N\ =\ T\delta\theta\)
\( \delta T\ =\ -\mu\delta N\)
and
\( \delta T\ =\ -\mu T\delta\theta\)
and hence by integration
\( \underline{\underline{F\ =\ M\text{g}e^{-\mu\alpha}}}\).
Area of square = \( 4a^{2}\)
Area of rectangle = \( 4a^{2}(1-x)\)
Area of triangle = \( 2a^{2}(x+y-1)\)
Area of trapezoid = \( 2a^{2}(1-x+y)\)
The weight of the cube is \( 8a^{3}\rho s\text{g}\), and it acts downward through C, the centre of mass. The hydrostatic upthrust is \( 4a^{3}(1-x+y)\rho\text{g}\) and it acts upward through the centre of buoyancy H. Here \( \rho\) is the density of the fluid, and \( \rho s\) is the density of the wood. We evidently must find the centre of mass. The hydrostatic upthrust is \( 4a^{3}(1-x+y)\rho\text{g}\) and it acts upward through the centre of buoyancy H. Here \( \rho\) is the density of the fluid, and \( \rho s\) is the density of the wood. We evidently must find the \( X'-\) coordinate of C and of H. Let’s first of all find the \( X-\) and \( Y-\) coordinates (see the next figure).
The \( X-\) and \( Y-\)coordinates of C are trivial and quite easy respectively:
\( X_{C}\ =\ a \qquad \qquad Y_{C}\ =\ a(1-2x)\)
You are going to have to work quite hard at it to find the \( X-\) and \( Y-\) coordinates of H, the centre of buoyancy, which is the centroid of the trapezoid. “After some algebra” you should findcentre of buoyancy, which is the centroid of the trapezoid. “After some algebra” you should find
\( X_{H}\ =\ \frac{2(1-x+2y)a}{3(1-x+y)} \qquad \qquad Y_{H}\ =\ \frac{2(2-4x+2y+2x^{2}-2xy-y^{2})a}{3(1-x+y)}\)
To find the \( X'-\) coordinates of C and of H, we use the usual formulas for rotation of axes, being sure to get it the right way round:
\( \begin{pmatrix}X'\\Y'\end{pmatrix}\ =\ \begin{pmatrix}\cos\theta & -\sin\theta\\ \sin\theta & \cos\theta\end{pmatrix}\begin{pmatrix}X\\Y\end{pmatrix}\),
together with
\( \tan\ \theta\ =\ x\ +\ y-1\).
Take moments about the axle (origin):
\( 8a^{3}\rho s\text{g}X'_{C}\ =\ 4a^{3}\rho\text{g}(1-x+y)\).
After a little more algebra, you should eventually arrive at
\( \underline{\underline{s=\frac{3-7x+2y+6x^{2}-3y^{2}-2x^{3}+y^{3}+3xy^{2}}{3(2-3x-y+2x^{2}+2xy)}}}\)
Let the radii of the cylinder and sphere be \( a\) and \( b\) respectively, and the mass of the sphere be \( M\). The angles \( \theta\) and \( \phi\) are related by \( a\theta\ =\ b\phi\). I have drawn the three forces on the sphere, namely its weight, the normal reaction of the cylinder on the sphere, and the frictional force on the sphere. The transverse acceleration of the centre of the sphere is \( (a+b)\ddot{\theta}\) and the centripetal acceleration is \( (a+b)\dot{\theta}^{2}\). The equations of motion are:centre of the sphere is \( (a+b)\ddot{\theta}\) and the centripetal acceleration is \( (a+b)\dot{\theta}^{2}\). The equations of motion are:
\[ M\text{g}\sin\theta\ -\ F\ =\ M(a+b)\ddot{\theta} \tag{1}\label{25.1} \]
and
\[ M\text{g}\cos\theta\ -\ N\ =\ M(a+b)\dot{\theta}^{2} \tag{2}\label{25.2} \]
The angular acceleration of the sphere about its centre is \( \ddot{\theta}\ +\ \ddot{\phi}\ =\ \left(1+\frac{a}{b}\right)\ddot{\theta}\), and its rotational inertia is \( \frac{2Mb^{2}}{5}\). The torque that is causing this angular acceleration is \( Fb\), and therefore the rotational equation of motion is
\[ Fb =\ \frac{2}{5}Mb^{2}\left(1+\frac{a}{b}\right)\ddot{\theta} \tag{3}\label{25.3} \]
Elimination of \( F\) between Equations (\( \ref{20.1}\)) and (\( \ref{20.3}\)) yields
\[ \ddot{\theta} = \frac{5\text{g}}{7(a+b)}\sin\theta. \tag{4}\label{20.4} \]
Write \( \ddot{\theta}\) as \( \dot{\theta}\frac{d\dot{\theta}}{d\theta}\) in the usual way and integrate with initial conditions \( \theta=\dot{\theta}=0\) or from energy considerations:
\[ \dot{\theta}^{2} =\frac{10\text{g}}{7(a+b)}(1-\cos\theta) \tag{5}\label{20.5} \]
Substitute for \( \ddot{\theta}\) and \( \dot{\theta}^{2}\) into Equation (\( \ref{20.2}\)) to obtain
\[ N =\ M\text{g}(17\cos\theta-10). \tag{6}\label{20.6} \]
This is zero, and the sphere leaves the cylinder, when \( \cos\theta\ =\ \frac{10}{17}\), \( \theta\ =\ 53^{\circ}58'\).
Surface density = \( \sigma \) g cm-2
Original sandwich:
Mass = 54\( \sigma\) g
\( x\)-coordinate of centre of mass = 3 cm centre of mass = 3 cm
\( y\)-coordinate of centre of mass = 4 cm centre of mass = 4 cm
Bite:
Mass = \( \frac{1}{2}\pi3^{2}\sigma\) =14.137 166 94\( \sigma\) g
Distance of centre of mass from hypotenuse = centre of mass from hypotenuse= \( \frac{4}{3\pi}\times 3\ =\ \frac{4}{\pi}\) = 1.273 239 545 cm
x-coordinate of centre of mass = 4.5 -centre of mass = \( 4.5\ -\ \frac{4}{\pi}\sin\theta=4.5-\frac{16}{5\pi}\) = 3.481 408 364 cm
y-coordinate of centre of mass = 6- centre of mass = \( 6\ -\ \frac{4}{\pi}\cos\theta\ =\ 6-\frac{12}{5\pi}\) = 5.236 056 273 cm
Remainder:
Mass = (54 - 14.137 166 94)\( \sigma\) = 39.862 833 06\( \sigma\) g
\( x\)-coordinate of centre of mass = \( \overline{x}\)centre of mass = \( \overline{x}\)
\( y\)-coordinate of centre of mass = \( \overline{y}\)centre of mass = \( \overline{y}\)
Moments:
39.862 833 06\( \overline{x}\) + 14.137 166 94 × 3.481 408 364 = 54 × 3. \(\underline{\underline{\overline{x} = 2.829\ 270\ 780\ \text{cm}}}\)
39.862 833 06\( \overline{y}\) + 14.137 166 94 × 5.236 056 273 = 53 × 4. \( \underline{\underline{\overline{y}\ =\ 3.561\ 638\ 436\ \text{cm}}}\)
This point is very close to the edge of the bite. The centre of the bite is at (4.5, 6), and its radius is 3. Its equation is thereforecentre of the bite is at (4.5, 6), and its radius is 3. Its equation is therefore
\( (x-4.5)^{2}\ +\ (y-6)^{2}\ =9,\) or \( x^{2}\ +\ y^{2}\ -9x\ -12y\ +\ 47.25=0\).
The line \( x\) = 2.829 270 780 cuts the circle where \( y^{2}-12y\ +\ 29.791\ 336\ 13\ =\ 0\). The lower of the two points of intersection is at \( y\) = 3.508 280 941 cm. The centre of mass is slightly higher than this and is therefore just inside the bite.centre of mass is slightly higher than this and is therefore just inside the bite.
Consider a portion of the band within the angle \( \delta\theta\). Its mass is \( \frac{m\delta\theta}{2\pi}\) When the band is spinning at angular speed \( \omega\) and its radius is \( r\), the centrifugal force on that portion is \( \delta F\ =\ \frac{mr\omega^{2}\delta\theta}{2\pi}\). (I leave it to the philosophers and the schoolteachers to debate as to whether there “really” is “such thing” as centrifugal force – I want to get this problem done, and I’m referring to a co-rotating frame.) The \( y\)-component of this force is \( \frac{mr\omega^{2}\delta\theta}{2\pi}\). Also, the tension in the band when its radius is \( r\) is \( T=2\pi k(r-a)\).
Consider the equilibrium of half of the band. The \( y\)-component of the centrifugal force on it is \( \frac{mr\omega^{2}}{2\pi}\int_{-\frac{\pi}{2}}^{+\frac{\pi}{2}}\cos\theta d\theta=\frac{mr\omega^{2}}{\pi}\). The opposing force is \( 2T\ =\ 4\pi k(r-a)\). Equating these gives \( \underline{\underline{\omega^{2}\ =\ \frac{4\pi^{2}k(r-a)}{mr}}}\).
Let the distance AB be \( l\) and the distance AC be \( c\). Let the mass of the rod be \( m\).
Consider an elemental portion \( \delta x\) of the rod at P at a distance \( x\) from A. Its weight is \( \frac{m\delta x}{l}\). When the rod is about to move, it will experience a frictional force \( \delta f\ =\ \frac{\mu m\text{g}\delta x}{l}\), which will be in the direction shown if P is to the left of C, and in the opposite direction if P is to the right of C. When the rod is just about to move (but has not yet done so) it is still in equilibrium. Consider the moment about A of the frictional forces on the rod. The clockwise moment of the frictional forces on AC must equal the counterclockwise moment of the frictional forces on CB. Thus
\( \frac{\mu m\text{g}}{l}\int_{0}^{c}x\ dx=\frac{\mu m\text{g}}{l}\int_{c}^{l}x\ dx\).
\( \therefore \qquad\qquad \underline{\underline{c=\frac{l}{\sqrt{2}}}}\).
The net force on the rod is
\( F\ -\ \frac{\mu m\text{g}}{l}\int_{0}^{c}dx\ +\ \frac{\mu m\text{g}}{l}\int_{c}^{l} dx\),
and this is zero, and therefore
\( \underline{\underline{F\ =\ \frac{\mu m\text{g}(2c-l)}{l}=(\sqrt{2}-1)\mu m\text{g}.}}\)
The cone slips when \( \tan\theta\ >\ \mu\).
It tips when C (the centre of mass) is to the left of M. centre of mass) is to the left of M.
The distance OC is \( \frac{h}{4}\). (See Chapter 1, Section 1.7). Therefore it tips when \( \tan\theta>4\frac{a}{h}\).
Thus it slips if \( \mu<4\frac{a}{h}\) and it tips if \( \mu<4\frac{a}{h}\).
When the block is just about to tip, the reaction of the table on the block acts at A and it is directed towards the point K, because, when three coplanar forces are in equilibrium they must act through a single point. The angle \( \lambda\) is given by \( \tan\lambda\ =\ \frac{a}{x}\). However, by the usual laws of friction, the block will slip as soon as \( \tan\lambda\ =\ \mu\). Thus the block will slip if \( \mu<\frac{a}{x}\), and it will tip if \( \mu>\frac{a}{x}\). Expressed otherwise, it will slip if \( x<\frac{a}{\mu}\) and it will tip if \( x>\frac{a}{\mu}\). The greatest possible value of \( x\) is \( 2a\); therefore the block will inevitably slip if \( \mu < \frac{1}{2}\)
+
When or if the cylinder is just about to tip, it is about to lose contact with the left hand peg. The only forces on the cylinder are the torque, the weight, and the reaction \( R\) of the right hand peg on the cylinder, which must be vertical and equal to \( m\text{g}\). But the greatest possible angle that the reaction \( R\) can make with the surface of the cylinder is the angle of friction \( \lambda\) given by \( \tan\ \lambda\ =\ \mu\). From geometry, we see that \( \sin\ \theta\ =\ k\), or \( \tan\ \theta\ =\ \frac{k}{\sqrt{1-k^{2}}}\). Thus the cylinder will slip before it tips if \( \mu\ <\ \frac{k}{\sqrt{1-k^{2}}}\) and it will tip before it slips if \( \mu\ >\ \frac{k}{\sqrt{1-k^{2}}}\).
If the cylinder tips (which it will do if \( \mu\ >\ \frac{k}{\sqrt{1-k^{2}}}\)), the clockwise torque t at that moment will equal the counterclockwise torque of the couple (\( R\) and \( m\text{g}\)), which is \( m\text{g}ka\). Thus the torque when the cylinder tips is
TIP:
\[ \tau\ =\ m\text{g}ak. \tag{1}\label{31.1} \]
When or if the cylinder is just about to slip, the forces are as shown above, in which I have resolved the reactions of the pegs on the cylinder into a normal reaction (towards the axis of the cylinder) and a frictional force, which, when slipping is about to occur, is equal to m times the normal reaction. The equilibrium conditions are
\( \mu(N_{1}\ +\ N_{2})\cos\theta\ +\ (N_{1}-N_{2})\sin\theta\ =\ 0\),
\( \mu(N_{1}\ -\ N_{2})\sin\theta\ -\ (N_{1}+N_{2})\cos\theta\ +\ m\text{g}=\ 0\)
and
\( \mu(N_{1}\ +\ N_{2})a\ =\ \tau\).
We can find \( N_{1}\ +\ N_{2}\) by eliminating \( N_{1}\ -\ N_{2}\) from the first two equations, and then, writing \( \sqrt{1-k^{2}}\) for \( \cos\theta\), we find that, when slipping is about to occur,
SLIP:
\[ \tau\ =\ m\text{g}a\ \times\ \frac{\mu}{1+\mu^{2}}\ \times\ \frac{1}{\sqrt{1-k^{2}}}. \tag{2}\label{31.2} \]
I have drawn below the functions
\( \frac{\tau}{m\text{g}a}\ =\ k\ \text{(tip) and}\ \frac{\tau}{m\text{g}a}\ =\ \frac{\mu}{1\ +\ \mu^{2}}\times\frac{1}{\sqrt{1-k^{2}}}\ \text{(slip)}\)
for \( k\ =\ 0.1,\ 0.3,\ 0.5,\ \frac{1}{\sqrt{2}}\) and \( 0.9\). The horizontal lines are the tip functions, and the curves are the slip functions. As long as \( \mu\ <\ \frac{k}{\sqrt{1-k^{2}}}\) the cylinder will slip. As soon as \( \mu\ <\ \frac{k}{\sqrt{1-k^{2}}}\) the cylinder will tip.
We’ll leave to the philosophers the question as to whether centrifugal force “really exists”, and we’ll work in a co-rotating reference frame, so that the car, when referred to that frame, is in static equilibrium under the six forces shown. Clearly, \( N_{1}\) and \( N_{2}\ =\ m\text{g}\) and \( F_{1}\ +\ F_{2}\ =\ \frac{mv^{2}}{R}\).
The car slips when \( F_{1}\ +\ F_{2}\ =\ \mu(N_{1}+N_{2})\); that is, when \( v\ =\ \sqrt{\mu\text{g}R}\).
The car tips when \(\frac{mv^{2}h}{R}\ =\ m\text{g}d;\) that is, when \( v\ =\ \sqrt{\frac{d\text{g}R}{h}}\).
That is, it will slip or tip according as to whether \( \mu\ < \frac{d}{h}\) or \( > \frac{d}{h}\).
For example suppose \( d\ =\ 60\ \text{cm},\ h\ =\ 60\ \text{cm},\ \text{g}\ =\ 9.8\ \text{m s}^{-2},\ R\ =\ 30\ \text{m},\ \mu\ =\ 0.8\).
In that case, \( \frac{d}{h}\ =\ 0.75\), so it will tip at \( v\ =\ 14.8\ \text{m s}^{-1}\ =\ 53.5\ \text{km hr}^{-1}\).
But if it rains, reducing \( \mu\) to 0.7, it will slip at \( v\ =\ 14.3\ \text{m s}^{-1}\ =\ 51.6\ \text{km hr}^{-1}\).
I have drawn in green the radial and transverse components of the acceleration of the centre of mass \( a\dot{\theta}^{2}\) and \( a\ddot{\theta}\) respectively. I have drawn in red the weight of the rod and the normal and frictional components of the force of the table on the rod at \( A\), \( N\) and \( F\) respectively.centre of mass \( a\dot{\theta}^{2}\) and \( a\ddot{\theta}\) respectively. I have drawn in red the weight of the rod and the normal and frictional components of the force of the table on the rod at \( A\), \( N\) and \( F\) respectively.
The following are the equations of motion:
Normal:
\[ ma\ddot{\theta}\ =\ m\text{g}\cos\theta\ -\ N. \tag{1}\label{33.1} \]
Lengthwise:
\[ ma\dot{\theta}^{2}\ =\ -m\text{g}\sin\theta\ +\ F. \tag{2}\label{33.2} \]
Rotation:
\[ k^{2}\ddot{\theta}\ =\ \text{g}a\cos\theta \tag{3}\label{33.3} \]
Here \( k\) is the radius of gyration about A, given by
\[ k^{2}\ =\ \frac{1}{3}l^{2}\ +\ a^{2}. \tag{4}\label{33.4} \]
From Equations (\( \ref{33.1}\)), (\( \ref{33.3}\)) and (\( \ref{33.4}\)), we obtain
\[ N\ =\ m\text{g}\cos\theta.\left(\frac{l^{2}}{l^{2}\ +\ 3a^{2}}\right). \tag{5}\label{33.5} \]
The space integral (see Chapter 6, Section 6.2) of Equation (\( \ref{33.3}\)), with initial condition \( \dot{\theta}\ =\ 0\) when \( \theta\ =\ 0\), results in
\[ \dot{\theta}^{2}\ =\ \frac{2\text{g}a}{k^{2}}\sin\theta. \tag{6}\label{33.6} \]
This can also be obtained by equation the loss of potential energy, \( m\text{g}a\sin\theta\) to the gain in kinetic energy, \( \frac{1}{2}mk^{2}\dot{\theta}^{2}\).
Combining this with Equations (\( \ref{33.2}\)) and (\( \ref{33.4}\)) leads to
\[ F\ =\ m\text{g}\sin\theta.\left(\frac{l^{2}\ +\ 9a^{2}}{l^{2}\ +\ 3a^{2}}\right). \tag{7}\label{33.7} \]
At the instant of slipping, \( F\ =\ \mu N\), and hence, from Equations (\( \ref{33.5}\)) and (\( \ref{33.7}\)) we find
\( \tan\theta\ =\ \frac{\mu}{1\ +\ 9(\frac{a}{l})^{2}}\).
I derive \( v^{2}\ =\ \text{g}x\ +\ \frac{\text{g}}{l}x^{2}\) by two different methods – one from energy considerations, the other from angular momentum considerations. First, energy.
If the table top is taken to be the zero level for potential energy, the initial potential energy was \( -\frac{1}{2}m.\text{g}.\frac{1}{4}l\ =\ -\frac{1}{8}m\text{g}l\).
When the length of the dangling portion is \( \frac{1}{2}l\ +\ x\) the potential energy is
\( -\left(\frac{\frac{1}{2}l\ +\ x}{l}\right)m.\text{g}.\frac{1}{2}\left(\frac{1}{2}l\ +\ x\right)\ =\ -\frac{m\text{g}}{2l}\left(\frac{1}{2}l\ +\ x\right)^{2}\ =\ -\frac{1}{8}m\text{g}l\ -\ \frac{1}{2}m\text{g}l\ -\ \frac{m\text{g}x^{2}}{2l}\).
The loss of potential energy is therefore \( \frac{1}{2}m\text{g}x\ +\ \frac{m\text{g}x^{2}}{2l}\).
This is equal to the gain in kinetic energy \( \frac{1}{2}mv^{2}\), and therefore
\( v^{2}\ =\ \text{g}x\ +\ \frac{\text{g}}{l}x^{2}\).
Another method:
Розглянемо точку А. Де завгодно буде робити, але я вибрав це відстань\( l\) below the level of the table and \( l\) to the left of the table edge. The moment of momentum (= angular momentum) of the chain about this point is \( mlv\ =\ ml\dot{x}\) and its rate of change is therefore \( mlv\ =\ ml\dot{x}\). The torque about A is \( \left(\mlv\ =\ ml\dot{x}\) and its rate of change is therefore \( mlv\ =ml\dot{x}\). The torque about A is \( \left(\frac{\frac{1}{2}l\ +\ x}{l}\right)m\text{g}l\ =\ \left(\frac{1}{2}l\ +\ x\right)m\text{g}\). These are equal, and so \( l\ddot{x}\ =\ \text{g}\left(\frac{1}{2}l\ +\ x\right)\). Write \( \ddot{x}\ =\ v\frac{dv}{dx}\) in the usual way, and integrate (with \( v=0\) when \( x=0\)) and the result \( v^{2}\ =\ \text{g}x\ +\ \frac{g}{l}x^{2}\) follows.
To find the relation between \( x\) and \( t\) we can use the energy Equation 9.2.9 for conservative systems
\( t\ =\ \sqrt{\frac{m}{2}}\int_{x_{0}}^{x}\frac{dx}{\sqrt{E-V(x)}}\).
Here \( x_{0}\ =\ 0\) and we have already seen that \( E\ -\ V(x)\ =\ \frac{m\text{g}}{2l}x^{2}\ +\ \frac{m\text{g}x}{2}\). Upon integrating this expression, we obtain, after a little algebra and calculus,
\[ \tag{1}\label{34.1} \]
The converse of this is the required expression
\[ \tag{2}\label{34.2} \]
Differentiation of this with respect to time produces the third required expression:
\[ \tag{3}\label{34.3} \]
You may verify from these last two equations, if you wish, that \( v^{2}\ =\ \text{g}x\ +\ \frac{\text{g}}{l}x^{2}\).
The chain falls completely off the table when \( x\ =\ \frac{1}{2}l\). That is (by using Equation (\( \ref{34.1}\))), at time \( \sqrt{\frac{l}{g}}\ln(2\ +\ \sqrt{3})\ =\ 1.317\sqrt{\frac{l}{\text{g}}}\).
If we express distances in units of \( l\), time in units of \( \sqrt{\frac{l}{\text{g}}}\) and therefore necessarily speeds in units of \( \sqrt{\text{g}l}\), Equations (\( \ref{34.2}\)) and (\( \ref{34.3}\)) become
\[ x\ =\ \frac{(e^{t}-1)^{2}}{4e^{t}}\ =\ \frac{1}{4}(e^{t}\ +\ e^{-t}-2)\ =\ \frac{1}{2}(\cosh t-1) \tag{4}\label{34.4} \]
\[ v\ =\ \frac{e^{2t}-1}{4e^{t}}\ =\ \frac{1}{2}\sinh\ t \tag{5}\label{34.5} \]
and we can get the acceleration by a further differentiation:
\[ a\ =\ \frac{1}{4}(e^{t}\ +\ e^{-t})\ =\ \frac{1}{2}\cosh\ t. \tag{6}\label{34.6} \]
We are pleased to note that, by the time that \( x\ =\ \frac{1}{2}l\) [i.e. when the chain completely leaves the table at time \( t\ =\ \ln(2\ +\ \sqrt{3})\sqrt{\frac{l}{\text{g}}}\) ], the acceleration is \( \text{g}\). The speed is then \( \sqrt{\frac{3}{4}l\text{g}}\ =\ 0.866\sqrt{l\text{g}}\).
The maximum overhang of book \( \bf{1}\) is \( d_{1}\ =\ w\).
The centre mass of \( \bf{1\ +\ 2}\)centre mass of \( \bf{1\ +\ 2}\) is at \( \frac{3w}{2}\) from the left hand side (LHS) of \( \bf{2}\), so \( d3\ =\ \frac{w}{3}\).
The distance of the centre of mass of \( \bf{1+2+3}\)centre of mass of \( \bf{1+2+3}\) is at \( \frac{5\text{w}}{2}\) from the LHS of \( \bf{3}\), so \( d3\ =\ \frac{w}{3}\).
Thus \( D\ =\ d_{1}\ +\ d_{2}\ +\ d_{3}\ =\ \left(1\ +\ \frac{1}{2}\ +\ \frac{1}{3}\right)\text{w}\ =1.8\dot{3}\text{w}\).
In a similar manner we find that, given \( n\ +\ 1\) books, the maximum overhang is
\( D\ =\ \left(1+\frac{1}{2}+\frac{1}{3}...\ ...+\frac{1}{n}\right)\text{w}\).
I do not know if there is a simple expression for the sum to \( n\) terms of this harmonic series. Please let me know if you know of one or can find one. Therefore I used a computer to solve
\( 1+\frac{1}{2}+\frac{1}{3}...\ ...+\frac{1}{n}\ =\ 10\)
by brute force. I got \( n = 12367\), so you would need 12368 books.
The harmonic series is divergent and has no finite limit, so there is no finite limit to the possible overhang.
You might wish to speculate on any practical limitations on constructing such a pile of books. For example, we have been assuming a uniform gravitational field – but this will no longer be valid once the overhang becomes comparable to the radius of Earth. This will, however, need quite a large number of books.
In the solution that follows, a prime \( \) will be used to denote differentiation with respect to \( x\), and \( p\ =\ y'\ =\ \frac{dy}{dx}\). I shall also make use of an auxiliary variable \( \phi\ =\ \sinh^{-1}p\). The initial conditions are \( y=0,\ x=a,\ p=0,\ \phi=0\). The final conditions are \( x=0,\ p=-\infty,\phi=-\infty,y\) to be determined.
At time \( t\), the \( y-\)coordinate of the Man is \( v\ t\). If \( (x,\ y)\) are the coordinates of the Dog at that time, the slope of the path taken by the Dog is
\[ p\ =\ -\frac{vt-y}{x}, \tag{1}\label{36.1} \]
so that
\[ v\ t\ =\ y-px. \tag{2}\label{36.2} \]
The speed of the Dog is
\[ A v =\ -\frac{ds}{dt}\ =\ -\sqrt{1+p^{2}}\frac{dx}{dt}. \tag{3}\label{36.3} \]
[This comes from \( ds\ =\ \sqrt{1+\left(\frac{dy}{dx}\right)^{2}}dx\). The minus sign is necessary because \( \left(\frac{dx}{dt}\right)\) is negative, and \( Av\), the speed (not velocity!) of the Dog is necessarily positive.]
Now \( \left(\frac{dx}{dt}\right)\ =\ -\frac{1}{t'}\) so Equation (\( \ref{eq:36.3}\)) can be written
\[ Avt'\ =\ -\sqrt{1+p^{2}} \tag{4}\label{36.4} \]
If we can eliminate \( t\) between Equations (\(\ref{36.2}\)) and (\( \ref{36.4}\)), we will obtain a relation between the slope \( p\) and \( x\), and hence potentially a relation between \( y\) and \( x\).
Differentiate Equation (\( \ref{eq:36.2)}\) with respect to \( x\) (recalling that \( y'=p\)):
\[ vt'\ =\ -p'x \tag{5}\label{36.5} \]
It is now simple to eliminate \( t'\) from Equations (\( \ref{36.4}\)) and (\( \ref{36.5}\)):
\[ Ap'x\ =\ \sqrt{1+p^{2}} \tag{6}\label{36.6} \]
On separating the variables and integrating, we obtain
\[ A\int\frac{dp}{\sqrt{1+p^{2}}}\ =\ \int\frac{dx}{x}. \tag{7}\label{36.7} \]
With initial conditions \( p=0\) when \( x=a\), this gives us
\[ A\sinh^{-1}p\ =\ \ln\left(\frac{x}{a}\right), \tag{8}\label{36.8} \]
or
\[ x\ =\ ae^{A\phi}, \tag{9}\label{36.9} \]
where
\[ \phi\ =\ \sinh^{-1}p. \tag{10}\label{36.10} \]
Equation (\( \ref{36.9}\)), with (\( \ref{36.10}\)), gives us the relation between \( x\) and the slope, \( p\). Note that \( p\) and hence \( f\) are negative, so that equation says that \( x<a\).
Our next task will be to find a relation between \( y\) and \( p\) (or between \( y\) and \( \phi\)).
From Equation (\( \ref{36.10}\)) we have
\[ dy\ =\ \sinh\phi\ dx, \tag{11}\label{36.11} \]
and from Equation (\( \ref{36.9}\)) we have
\[ dx\ =\ aAe^{A\phi}d\phi. \tag{12}\label{36.12} \]
From these we obtain the differential relation between \( y\) and \( \phi\):
\[ dy\ =\ aAe^{A\phi}\sinh\phi d\phi, \tag{13}\label{36.13} \]
or
\[ dy\ =\ \frac{1}{2}aA(e^{(A+1)\phi}\ -\ e^{(A-1)\phi})d\phi. \tag{14}\label{36.14} \]
Integrate this, with initial condition \( \phi\ =\ 0\) when \( y\ =\ 0\), to obtain
\[ y\ =\ \frac{1}{2}aA\left(\frac{\left(\frac{x}{a}\right)^{1+1/A}}{A+1}-\frac{e^{(A-1)\phi}}{A-1}+\frac{2}{A^{2}-1}\right). \tag{15}\label{36.15} \]
Equation (\( \ref{36.9}\)) and (\( \ref{36.15}\)) are parametric equations to the path of the Dog, though it is easy to eliminate \( \phi\) and write \( y\) explicitly as a function of \( x\):
\[ y\ =\ \frac{1}{2}aA\left(\frac{e^{(A+1)\phi}}{A+1}-\frac{e^{(A-1)\phi}}{A-1}+\frac{2}{A^{2}-1}\right). \tag{16}\label{36.16} \]
The figure was drawn for \( a=1,\ A=2,\) for which Equation (\( \ref{36.16}\)) reduces to
\[ y\ =\ \frac{1}{3}[x^{\frac{1}{2}}(x-3)\ +\ 2]. \tag{17}\label{36.17} \]
The distance walked by the Man is found by putting \( \phi=-\infty\) in Equation \( \ref{36.15}\). Thus
\[ y\ =\ \frac{aA}{A^{2}-1}, \tag{18}\label{36.18} \]
and the time taken is
\[ t\ =\ \frac{aA}{v(A^{2}-1)} \tag{19}\label{36.19} \]
Let \( l\) be the length of the string.
Kinetic energy of the upper mass = \( \frac{1}{2}(mr^{2})\omega^{2}\ +\frac{1}{2}m\dot{r}^{2}\).
Kinetic energy of the lower mass = \( \frac{1}{2}m\dot{r}^{2}\)
Potential energy of the lower mass = \( m\text{g}(l-r)\).
Total energy of the system =
\[ \frac{1}{2}(mr^{2})\omega^{2}\ + m\dot{r}^{2}\ -\ m\text{g}(l-r). \tag{1}\label{37a.1} \]
Initial total energy of the system =
\[ \frac{1}{2}(ma^{2})\omega_{0}^{2}\ + m\dot{r}^{2}\ -\ m\text{g}(l-a). \tag{2}\label{37a.2} \]
Energy is conserved and therefore, by equating (\( \ref{37a.1}\)) and (\( \ref{37a.2}\)), we obtain
\[ \dot{r}^{2}\ =\ \text{g}(a-r)\ +\ \frac{1}{2}a^{2}\omega_{0}^{2}\ -\ \frac{1}{2}r^{2}\omega^{2}. \tag{3}\label{37a.3} \]
Angular momentum is also conserved, and therefore
\[ r^{2}\omega\ =\ a^{2}\omega_{0} \tag{4}\label{37a.4} \]
On elimination of \( r\) between Equations (\( \ref{37a.3}\)) and (\( \ref{37a.4}\)) we obtain, after some algebra,
\[ \frac{\dot{r}^{2}}{\text{g}a}\ =\ 1\ +\ \frac{a\omega_{0}^{2}}{2\text{g}}\left(1-\frac{\omega}{\omega_{0}}\right)\ -\sqrt{\frac{\omega_{0}}{\omega}}. \tag{5}\label{37a.5} \]
If \( a\omega_{0}^{2}\ =\ \text{g}\) it is trivial to show that
\[ \frac{\dot{r}^{2}}{\text{g}a}\ =\ \frac{3}{2}\ -\frac{1}{2}\Omega\ -\frac{1}{\sqrt{\Omega
}}. \tag{6}\label{37a.6} \]
Algebra and calculus show that \( \frac{3}{2}\ -\frac{1}{2}\Omega\ -\frac{1}{\sqrt{\Omega
}}\) is negative for all positive \( \Omega\) except for \( \Omega\ =\ 1\), when it reaches a maximum value of zero.
If \( a\omega_{0}^{2}\ =\ 2\text{g}\) and \( \Omega\ =\ \frac{\omega}{\omega_{0}}\) it is trivial to show that
\[ \frac{\dot{r}^{2}}{\text{g}a}\ =\ 2\ -\ \Omega\ -\frac{1}{\sqrt{\Omega}} \tag{7}\label{37a.7} \]
Algebra and calculus show that \( 2\ -\ \Omega\ -\frac{1}{\sqrt{\Omega}}\) reaches a maximum value for \( \Omega\ =\ \frac{\omega}{\omega_{0}}\ =\ \frac{1}{2^{\frac{2}{3}}}\ =\ 0.629\ 961\) at which time \( \frac{\dot{r}^{2}}{(\text{g}a)}\ =\ 0.110\ 118\). That is, when \( \dot{r}\ =\ 0.331\ 841\sqrt{\text{g}a}\) Equation (\( \ref{37a.4}\)) (conservation of angular momentum) shows that \( r\ =\ \frac{a}{\sqrt{\Omega}}\ =\ \sqrt[3]{2a}\ =\ 1.259\ 921a\).
Solution of \( 2\ -\ \Omega\ -\ \frac{1}{\sqrt{\Omega}}=0\) shows that the speed is zero when \( \Omega\ =\ 1\) (the initial condition) and when \( \) (the equilibrium value). Equation (\( \ref{37a.4}\)) (conservation of angular momentum) shows that \( r\ =\ \frac{a}{\sqrt{\Omega}}\ =\ 1.618\ 034a\).
If \( a\omega_{0}^{2}\ =\ \frac{1}{2}\text{g}\) and \( \Omega\ =\frac{\omega}{\omega_{0}}\) it is trivial to show that
\[ \frac{\dot{r}^{2}}{\text{g}a}\ =\ \frac{5}{4}\ -\frac{1}{4}\Omega\ -\frac{1}{\sqrt{\Omega}} \tag{8}\label{37.8} \]
Algebra and calculus show that \( \frac{5}{4}\ -\frac{1}{4}\Omega\ -\frac{1}{\sqrt{\Omega}}\) reaches a maximum value for \( \Omega\ =\ \frac{\omega}{\omega_{0}}\ =\ 2^{\frac{2}{3}}\ =\ 1.587\ 401\) at which time \( \frac{\dot{r}^{2}}{(\text{g}a)}=0.059449\). That is, when \( \dot{r}\ =\ -0.243\ 822\sqrt{\text{g}a}\) Equation (\( \ref{37a.4}\)) (conservation of angular momentum) shows that \( r\ =\ \frac{a}{\sqrt{\Omega}}\ =\ \frac{a}{\sqrt[3]{2}}\ =\ 0.793\ 701a\).
Solution of \( \frac{5}{4}\ -\frac{1}{4}\Omega\ -\frac{1}{\sqrt{\Omega}}\) shows that the speed is zero when \( \Omega\ =\ 1\) (the initial condition) and when \( \Omega\ =\ \frac{\omega}{\omega_{0}}\ =\ 2.438\ 447\) (the equilibrium value). Equation (\( \ref{37a.4}\)) (conservation of angular momentum) shows that \( r\ =\ \frac{a}{\sqrt{\Omega}}\ =\ 0.640\ 388a\).
How much further can we go with this question? By elimination of \( r\) between Equations (\( \ref{37a.3}\)) and (\( \ref{37a.4}\)) we obtained a relation between \( \dot{r}\) and \( \omega\). By elimination of \( \omega\) between Equations (\( \ref{37a.3}\)) and (\( \ref{37a.4}\)) we can get a relation between \( \dot{r}\) and \( r\). It will be of the form
\[ \dot{r}\ =\ \sqrt{A\ -\ \text{g}r\ -\ \frac{B}{r^{2}}}, \tag{9}\label{37a.9} \]
where \( A\ =\ \text{g}a\ +\ \frac{1}{2}a^{2}\omega_{0}^{2}\) and \( B\ =\ a^{4}\omega_{0}^{4}\). If you can integrate this, you then get a relation between \( r\) and \( t\). I haven’t given much though as to whether you can get integrate Equation (\( \ref{37a.9}\)) analytically (if anyone manages it, please let me know), but at least a numerical integration will certainly be possible.
In another variation of the question, you can start with an equilibrium situation in which \( a\omega_{0}^{2}\ =\ \text{g}\) and then add an extra mass \( m\) (or \( M\), if you want to make it more general) and then follow the motion from there. I leave that to you.
Let’s look at the rod from above when it is twisted in the horizontal plane through a small angle \( \theta\).
Each of the points where the threads are attached to the rod is displaced horizontally through a distance \( \frac{1}{2}D\theta\) (Since \( \theta\) is small and \( D\ <<L\) we can neglect the slight vertical rise in the position of the rod.) Each thread is now displaced from the vertical by an angle \( \phi\) given by
The tension \( T\) in each thread is \( \frac{1}{2}m\text{g}\cos\phi\) which, to first order in \( \phi\), is just \( \frac{1}{2}m\text{g}\).
The horizontal component of each of these forces is \( \frac{1}{2}m\text{g}\sin\phi\) which, to first order in \( \phi\), is \( \frac{1}{2}m\text{g}\phi\).
Therefore the rod experiences a restoring torque equal to \( \frac{1}{2}m\text{g}D\phi\). But \( \phi\ =\ \frac{\frac{1}{2}D\theta}{L}\) and therefore the restoring torque is \( \frac{m\text{g}D^{2}\theta}{4L}\).
The equation of motion is therefore
\( I\ddot{\theta}\ =\ -\frac{mgD^{2}}{4L}\theta\)
and consequently the period \( P\) of small oscillations is
\( P\ =\ 2\pi\sqrt{\frac{4LI}{mgD^{2}}}\ =\ \frac{4\pi}{D}\sqrt{\frac{LI}{m\text{g}}}\).
If the rod is uniform and of length \( 2l\), its moment of inertia is \( \frac{1}{3}ml^{2}\) and in that case the period of small oscillations is
\( P\ =\ \frac{4\pi l}{D}\sqrt{\frac{L}{3\text{g}}}\).
There is no need to remind the reader to check the dimensions of these equations.
When the yo-yo has fallen through a distance \( x\), it has lost potential energy \( Mgh\), and it has gained translational kinetic energy \( \frac{1}{2}mv^{2}\) and gained rotational kinetic energy \( \frac{1}{2}I\omega^{2}\) where \( \omega\ =\ \frac{v}{a}\). Therefore \( Mgx\ =\ \frac{1}{2}Mv^{2}\ +\ \frac{1}{2}\ I\left(\frac{v}{a}\right)^{2}\) from which
\( v^{2}\ =\ 2\ \cdot\ \frac{Ma^{2}\text{g}}{Ma^{2}\ +\ I}\ \cdot\ x\).
Thus, from the usual equations for constant linear acceleration, the acceleration is
\( \frac{Ma^{2}}{Ma^{2}\ +\ I}\times\text{g}\).
The net downward force is \( M\text{g}-P\) where \( P\) is the tension in the string. This is equal to \( M\) times the acceleration, from which we obtain
\( P\ =\ \frac{1}{Ma^{2}+1}\times M\text{g}.\)
I have drawn four forces on the yo-yo. Its weight \( M\text{g}\). The tension \( P\) in the string. The normal reaction \( N\) of the table on the yo-yo. And the frictional force \( F\) of the table on the yo-yo. As long as the yo-yo is in contact with the table and there is no vertical acceleration, we must have \( P\ +\ N\ =\ M\text{g}\).
Let us suppose that there is no slipping between the yo-yo and the table, so that the yo-yo rolls to the left. We note that there is a net force \( F\) to the left, and a net counterclockwise torque about C equal to \( Pa-Fb\). Thus the yo-yo accelerates to the left at a rate \( \frac{F}{M}\) it and experiences a counterclockwise angular acceleration \( (Pa-Fb)\frac{a}{I}\). If there is no slipping, these must be related by \( \frac{F}{M}=b\times\frac{Pa-Fb}{I}\). Thus, if there is no slipping,
\[ F\ =\ \left(\frac{Mab}{I\ +\ Mb^{2}}\right)P. \tag{1}\label{40a.1} \]
The linear acceleration to the left must be \( \frac{F}{M}\), or
\[ \underline{\underline{\frac{abP}{I+Mb^{2}}}} \tag{2}\label{40a.2} \]
_________________________
Alternative derivation:
There is a net counterclockwise torque about A equal to \( Pa\). The moment of inertia with respect to A is \( I\ +\ Mb^{2}\). Therefore there is an angular acceleration about A equal to \( \frac{Pa}{I\ +\ Mb^{2}}\). Therefore the linear acceleration of C to the left is \( \underline{\underline{\frac{abP}{I\ +\ Mb^{2}}}}\) and the frictional force \( F\) is \( M\) times this, or \( F\ =\ \left(\frac{Mab}{I\ +\ Mb^{2}}\right)P\).
End of Alternative Derivation.
__________________________
However, if the yo-yo is just about to slip, \( F\ =\ \mu N\ = \ \mu(M\text{g}-P)\). Upon substitution of this into Equation (\( \ref{40a.1}\)), we see that the yo-yo will just slip if
\[ P\ =\ \frac{\mu M\text{g}(I\ +\ Mb^{2})}{\mu(I\ +\ Mb^{2})\ +\ Mab}. \tag{3}\label{40a.3} \]
That is, the yo-yo will roll to the left without slipping if
\[ \underline{\underline{\mu\ >\ \frac{MabP}{(M\text{g}-P)(I+Mb^{2})}}} \tag{4}\label{40a.4} \]
Its linear acceleration is then given by Equation (\( \ref{40a.2}\)), namely
\[ \underline{\underline{\frac{abP}{I+Mb^{2}}}} \tag{2}\label{40a.2b} \]
On the other hand, the yo-yo will rotate counterclockwise with no rolling if
\[ \underline{\underline{\mu\ <\ \frac{MabP}{(M\text{g}-P)(I+Mb^{2})}}} \tag{5}\label{40a.5} \]
The sum of the counterclockwise moments of the forces about C is then \( Pa-Fb\) where \( F=\mu N=\mu(M\text{g}-P)\). The counterclockwise angular acceleration about C is
\[ \frac{Pa-Fb}{I}\ =\ \underline{\underline{\frac{P(a-\mu b)-\mu M\text{g}}{I}}}. \tag{6}\label{40a.6} \]
I have drawn the four forces on the yo-yo. Its weight \( M\text{g}\). The normal reaction of the table on the yo-yo, which is also of magnitude \( M\text{g}\). The tension \( P\) in the string. And the frictional force \( F\) of the table on the yo-yo.
At this point it may not be immediately obvious whether \( F\) acts to the left or the right. For example, let us suppose that the coefficient of friction is zero. The force \( P\) will result in a translation of the yo-yo to the right together with a clockwise rotation of the yo-yo. So, in which direction does the point A on the circumference of the yo-yo move - to the left or the right? It is hard to say, but one might suppose, qualitatively, that, if the moment of inertia is large, the induced rotation will be sluggish, so that A moves to the right. Whereas if \( I\) is small, the induced rotation will be rapid, and A will move to the left in spite of the translational motion of the centre of mass to the right. From this we might conclude that, if \( \mu\neq 0\), \( F\) will act to the right if \( I\) is small, and \( F\) will act to the left if \( I\) is large. The following analysis shows that this qualitative expectation is correct.centre of mass to the right. From this we might conclude that, if \( \mu\neq 0\), \( F\) will act to the right if \( I\) is small, and \( F\) will act to the left if \( I\) is large. The following analysis shows that this qualitative expectation is correct.
(The reader might find some of the Problems in Section 8.2 of Chapter 8 to be helpful at this point, particularly Problem 2.5.)
For the time being, I have drawn \( F\) as if acting towards the left.
Let us suppose there is no slipping and that the yo-yo rolls.
The sum of the clockwise moments of the forces about A is \( P(a\ +\ b)\) and the moment of inertia about A is \( I\ +\ Mb^{2}\). The yo-yo therefore undergoes an initial clockwise angular acceleration about A equal to \( \frac{P(a\ +\ b)}{I+Mb^{2}}\) and, therefore (if there is no slipping), an initial linear acceleration of C to the right equal to
\[ \underline{\underline{\frac{Pb(a\ +\ b)}{I\ +\ Mb^{2}}}}. \tag{1}\label{40b.1} \]
The above linear acceleration must equal \( \frac{(P-F)}{M}\) from which we obtain
\[ F\ =\ \left(\frac{I-Mab}{I+Mb^{2}}\right)P \tag{2}\label{40b.2} \]
This shows that the frictional force \( F\) acts to the left, as shown, if \( I\ >\ Mab\) but if \( I<Mab\) the frictional force \( F\) acts to the right. This is in agreement with our qualitative expectations, namely that \( F\) will act to the left if \( I\) is large, and to the right if \( I\) is small.
Let is consider three cases in turn: \( I>Mab,\ I<Mab\) and \( I=Mab\).
(i) \( I>Mab\)
In this case, \( F\) acts to the left, as drawn. Provided \( F<\mu M\text{g}\) there will be no slipping at A, and the yo-yo will roll to the right without slipping. On recalling Equation (\( \ref{40b.2}\)), we see that the yo-yo will roll to the right without slipping, with a linear acceleration given by Equation (\( \ref{40b.1}\)) if
\[ \underline{\underline{\mu>\left(\frac{I-Mab}{I+Mb^{2}}\right)\left(\frac{P}{M\text{g}}\right)}}. \tag{3}\label{40b.3} \]
The linear acceleration to the right is given by Equation (\( \ref{40b.1}\)), namely
\[ \underline{\underline{\frac{Pb(a\ +\ b)}{I\ +\ Mb^{2}}}}. \tag{1}\label{40b.1b} \]
However, if
\[ \underline{\underline{\mu<\left(\frac{I-Mab}{I+Mb^{2}}\right)\left(\frac{P}{M\text{g}}\right)}}. \tag{4}\label{40b.4} \]
slipping occurs at A. The frictional force is the no longer given by Equation (\( \ref{40b.2}\)), but is given by
\[ F=\mu M\text{g}, \tag{5}\label{40b.5} \]
and it acts to the left.
(We are concerned in this problem with the initial motion. Once motion is underway, \( \mu\) has to be replaced with the smaller coefficient of kinetic friction.)
The net force to the right is then \( P-\mu M\text{g}\) so the linear acceleration of C to the right is
\[ \underline{\underline{\frac{P-\mu M\text{g}}{M}}}. \tag{6}\label{40b.6} \]
Because of condition (\( \ref{40b.4}\)), this is necessarily positive.
The net clockwise moment of the forces about the centre of mass C is \( Pa\ +\ \mu M\text{g}b\). The yo-yo therefore undergoes a clockwise angular acceleration about C ofcentre of mass C is \( Pa\ +\ \mu M\text{g}b\). The yo-yo therefore undergoes a clockwise angular acceleration about C of
\[ \underline{\underline{\frac{Pa\ +\ \mu M\text{g}b}{I}}}. \tag{7}\label{40b.7} \]
The linear acceleration to the right of the point A on the circumference of the yo-yo is \( \frac{P\ -\ \mu M\text{g}}{M}\ -\ b\ \times\ \frac{Pa\ +\ \mu M\text{g}b}{I}\), and, because of condition (\( \ref{40b.4}\)), some algebra will show that this is necessarily positive, as expected.
(ii) \( I<Mab\)
In this case, \( F\) acts to the right, and the linear acceleration is \( \frac{(P\ +\ F)}{M}\). Provided that
\[ \underline{\underline{\mu\ >\ \left(\frac{Mab-I}{Mb^{2}+I}\right)\left(\frac{P}{M\text{g}}\right)}}, \tag{8}\label{40b.8} \]
the yo-yo will roll to the right with a linear acceleration given by Equation (\( \ref{40b.1}\)), namely
\[ \underline{\underline{\frac{Pb(a\ +\ b)}{I\ +\ Mb^{2}}}}. \tag{1}\label{40b.1c} \]
However, if
\[ \underline{\underline{\mu\ >\ \left(\frac{Mab-I}{Mb^{2}+I}\right)\left(\frac{P}{M\text{g}}\right)}}, \tag{9}\label{40b.9} \]
slipping occurs at A. The frictional force is then given by
\[ F\ =\ \mu M\text{g}, \tag{10}\label{40b.10} \]
and it acts to the right.
The net force to the right is then \( P\ +\ \mu M\text{g}\), so the linear acceleration to the right is
\[ \underline{\underline{\frac{P\ +\ \mu M\text{g}}{M}}}. \tag{11}\label{40b.11} \]
The net clockwise moment of the forces about the centre of mass C is \( \). The yo-yo therefore undergoes a clockwise angular acceleration about C ofcentre of mass C is \( \). The yo-yo therefore undergoes a clockwise angular acceleration about C of
\[ \underline{\underline{\frac{Pa\ -\ \mu M\text{g}b}{I}}}. \tag{12}\label{40b.12} \]
The linear acceleration to the left of the point A on the circumference of the yo-yo is \( b\times\frac{Pa-\mu M\text{g}b}{I}-\frac{P+\mu M\text{g}}{M}\), and, because of condition (\( \ref{40b.8}\)), some algebra will show that this is necessarily positive, as expected.
\( I=Mab\)
In this case, \( F\) is zero. Whatever the coefficient of friction, even zero, the yo-yo will undergo a linear acceleration \( \frac{P}{M}\) to the right (Verify that this is consistent with Equation (\( \ref{40b.1}\)) ), and a clockwise angular acceleration about C equal to \( \frac{Pa}{I}\). The linear acceleration to the right of the point A on the circumference of the yo-yo is
\( \frac{P}{M}\ -b\times\frac{Pa}{I}\)
which is zero. The initial linear velocity of the point A is therefore zero.
I have drawn the four forces on the yo-yo. Its weight \( M\text{g}\). The normal reaction of the table on the yo-yo, which is also of magnitude \( M\text{g}\). The tension \( P\) in the string. And the frictional force \( F\) of the table on the yo-yo. On this occasion (unlike in Problem 40 (b)) there is no question about the direction of \( F\), which is towards the left.
Let us suppose there is no slipping.
The sum of the clockwise moments of the forces about A is \( \frac{P(b-a)}{I\ +\ Mb^{2}}\) and the moment of inertia about A is \( I\ +\ Mb^{2}\). The yo-yo therefore undergoes an initial clockwise angular acceleration about A equal to \( \frac{P(b-a)}{I\ +\ Mb^{2}}\) and therefore (if there is no slipping) an initial linear acceleration to the right equal to
\[ \underline{\underline{\frac{Pb(b-a)}{I\ +\ Mb^{2}}}}. \tag{1}\label{40c.1} \]
Additional string therefore becomes wrapped around the axle. (Yes, it really does! I tried it!)
The above linear acceleration must equal \( \frac{(P-F)}{M}\) from which we obtain
\[ F\ =\ \left(\frac{I\ +\ Mab}{I\ +\ Mb^{2}}\right)P. \tag{2}\label{40c.2} \]
Provided \(F < \mu Mg\),there will be no slipping at A, and the yo-yo will roll to the right without slipping. Thus the yo-yo will roll to the right without slipping, with a linear acceleration given by equation (1) if
\[\underline{\mu> \frac{\left( \frac{I+Mab}{I+Mb^2}\right)}{\left( \frac{P}{Mg}\right)}.} \tag{2}\label{40c.3} \]
\[ \underline{\mu < \frac{\left( \frac{I+Mab}{I+Mb^2}\right)}{\left( \frac{P}{Mg}\right)}}, \label{40c.4} \]
\[ F = \mu Mg, \tag{2}\label{40c.5} \]
\[ \underline{\frac{P- \mu Mg}{M}}.\label{40c.6} \]
\[\underline{\frac{Pa- \mu Mgb}{I}} \label{40c.7} \]
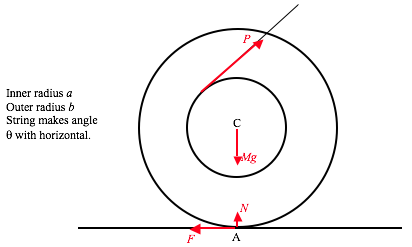
\[ \frac{Pb(a + b \cos \theta}{I+Mb^2} \tag{1}\label{40d.1} \]
\[F = \left(\frac{I \cos \theta - Mab}{I+Mb^2}\right)P. \tag{2}\label{40d.2} \]
\[ \frac{P(b \cos \theta - a)}{I + Mb^2}. \tag{1}\label{40e.1} \]
\[ \frac{P(b-\cos \theta - a)}{I + Mb^2}. \tag{2}\label{40e.2} \]
\[ F = \left(\frac{I \cos \theta + Mab}{I + Mb^2}\right)P, \tag{3}\label{40e.3} \]
\[ \frac{P(a-b\cos\theta}{I+Mb^2}. \tag{4}\label{40e.4} \]
\[ \frac{Pb(a-b\cos\theta}{I+Mb^2} \tag{5}\label{40e.5} \]
\[ F = \left(\frac{I\cos \theta + Mab}{I + Mb^2}\right)P, } \label{40e.6} \]
\(3 m \overline{x} = 2 ma + m \times \frac{4}{3} a \)
\(3 m \overline{y} = 2 m \times \frac{1}{2}a + m \times{4}{3} a. \)
\(\overline{x} = \frac{10}{9} a \qquad \overline{y} = \frac{7}{9}a. \)
\(\frac{1}{2}\begin{bmatrix}x_1 & x_2 & x_3 \\ y_1 & y_2 & y_3 \\ 1 & 1 &1 \end{bmatrix}.\)
\(\frac{1}{3}(2m)(\frac{1}{2} a)^2 = \frac{1}{6} ma^2. \)
\( = \frac{1}{3}(2m)a^2 = \frac{2}{3} ma^2.\)
= 0.
\( A_{rect} = \frac{1}{6} ma^2 + 2m(\frac{5}{18}a)^2 = \frac{28}{81}ma^2. \)
\( B_{rect} = \frac{2}{3} ma^2 + 2m(\frac{1}{9} a)^2 = \frac{65}{81} ma^2\)
\( H_{rect} = 0 + 2m(\frac{1}{9}a)(\frac{5}{18}a) = \frac{5}{81} ma^2. \)
\( = \frac{1}{6} ma^2 - m(\frac{1}{3}a)^2 = \frac{1}{18}ma^2. \)
\( = \frac{1}{6} (m)(2a)^2 - m( \frac{1}{3} \times 2a)^2 = \frac{2}{9}ma^2.\)
\( + \frac{1}{36}m(2a)(a) = +\frac{1}{18} ma^2.\)
\( A_{tria} = \frac{1}{18} ma^2 + m(\frac{5}{8} a)^2 = \frac{59}{162}ma^2. \)
\( B_{tria} = \frac{2}{9} ma^2 + m(\frac{2}{9} a)^2 = \frac{22}{81}ma^2.\)
\(H_{tria} = \frac{1}{18}ma^2 + m(-\frac{2}{9}a)(-\frac{5}{9}a) =\frac{29}{162}ma^2. \)
\(A = \frac{26}{81}ma^2 + \frac{59}{162}ma^2 = \frac{37}{51}ma^2.\)
\(B = \frac{56}{81}{ma^2+\frac{22}{81}}ma^2 = \frac{26}{27}ma^2.\)
\(H = \frac{5}{81}ma^2+\frac{29}{162}m= \frac{13}{54}ma^2.\)
\( \theta = \) 30º.009 180 and 120º.009 180
\( A \cos^2 \theta - 2 H \sin \theta \cos \theta + b \sin^2 \theta. \)
\(A_0\) = 0.546142ma2 , \(B_0\)= 1.102006ma2.
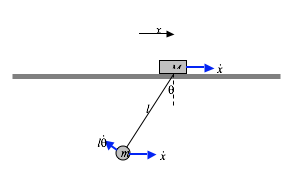
\(T = \frac{1}{2} Mx^2 + \frac{1}{2}m(\dot{x}^2 + l^2 \dot{\theta}^2 +2l\dot{\theta}\dot{x} \cos \theta\)
\(V = \text{constant} - mgl \cos \theta. \)
\( (M+ m)\ddot{x} + ml(\ddot{\theta} \cos \theta - \dot{\theta}^2 \sin \theta) = 0,\)
\(l \ddot{\theta} + \ddot{x} + g \theta = 0.\)
\( \ddot{\theta} = - \frac{(M+m)g}{Ml}\theta \)
\(\underline{\underline{2 \pi = \sqrt{\frac{Ml}{M+m}g}}}.\)
\( \frac{1}{k} \frac{dr}{d \alpha} = - \frac{1}{2} \sin \alpha \sin 2 \alpha + 2 \cos^2 \frac{1}{2} \alpha \cos 2 \alpha \)
\(= - \sin^2 \alpha \cos \alpha + (1 + \cos \alpha) (2 \cos^2 \alpha -1) = 0. \)
\( \underline{\underline{3c^2 + 2c^2 - 2c - 1 = 0}}\)
The condition for stability, from Chapter 16 Section 16.9, Equation 16.9.5 is that
\( \frac{Ak^{2}}{V}>\text{HC}\).
\( k^{2}\) for a filled circle of radius \( a\) is \( \frac{1}{4}a^{2}\). If the length of the cylinder is \( l\), the volume immersed is \( Asl\), so the left hand side of the inequality is \( \frac{a^{2}}{4sl}\).
The depth of the centre of mass is \( l(s-\frac{1}{2})\) and the depth of the centre of buoyancy is \( \frac{1}{2}ls\), so that \( \text{HC}\ =\ \frac{1}{2}l(1-s)\). The condition for stability is, then, \( \frac{a^{2}}{4sl}>\frac{1}{2}l(1-s)\).
With \( L\ =\frac{l}{(2a)}\), this gives, for the condition for stability,
\( \underline{\underline{L\ <\ \frac{1}{\sqrt{8s(1-s)}}}}\)
This function is least for \( s=\frac{1}{2},\ L\ <\ \frac{1}{\sqrt{2}}=0.707\). For any length less than this, the system is stable for any density. With \( L=1\), the inequality can be written \( 8s^{2}-8s+1>0\), so that \( s\) must be less than 0.146 or greater than 0.854.
Before doing the problem, let’s just have a look at the “interesting” property of a (4, 5, 6) triangle
Calculate \( A\) by the cosine rule: \( 16\ =\ 25\ +\ 36\ -60\cos A\), hence \( \cos A\ =\ \frac{3}{4}\).
Calculate \( C\) by the cosine rule: \( 36\ =\ 16\ +\ 25\ -40\cos C\), hence \( \cos C\ =\ \frac{1}{8}\).
But \( \cos 2A\ =\ 2\cos^{2}\ A\ -1\ =\frac{1}{8}\). Therefore \( C\ =\ 2A\).
The external angle at B is \( 3A\).
The angles are \( A\ =\ 41^{\circ}.4096 \quad C\ =\ 82^{\circ}.8192 \quad B\ =\ 55^{\circ}.7711 \quad \left(\cos B=\frac{9}{16}\right)\)
Supplement of \( B\ =\ 124^{\circ}.2289\)
It is not the case that a triangle with one angle equal to twice another one is necessarily a (4, 5, 6) triangle.
After that diversion, let’s move on to the given problem - except that we’ll generalize it to make the length of the rod \( 2l\), and the lengths of the strings \( a\) and \( b\).
The only physics involved is to recall that, if three coplanar forces are in equilibrium, they must be concurrent at a point - in this case the point C. This means that C must be vertically above the mid-point of the rod.
After that, there is no more physics; the rest is “just” geometry. All we have to do is to find \( \theta\) in terms of \( a\) and \( b\).
If C is to be directly above the mid-point, then AN = MB. That is:
\( b\cos(A+\theta)\ =\ a\cos(B-\theta)\).
This quickly results in
\( \tan\theta=\frac{b\cos A\ -\ a\cos B}{b\sin A\ +\ a\sin B}\).
In our particular example, \( \frac{a}{b}\ =\ \frac{4}{5}\ =\ 0.8,\ B\ =\ 180^{\circ}\ -\ 3A\), and \( A\ =\ 41^{\circ}.4096\).
Thus
\( \tan\theta\ =\ \frac{\cos A\ +\ 0.8\cos3A}{\sin A\ +\ 0.8\sin3A}\),
and
\( \underline{\theta\ =\ 12^{\circ}.78}\)
If the weight of the rod is \( m\text{g}\), I’ll leave it to you to work out the tensions in the strings.
The only physics involved is to recall that, if three coplanar forces are in equilibrium, they must be concurrent at a point - in this case the point C. This means that C must be vertically above the mid-point. Also, since the planes are smooth, the forces at A and B are perpendicular to the planes.
The rest is geometry - almost the same as in Problem 45, except that in this problem we are given the angles \( \alpha\) and \( \beta\) rather than the lengths \( a\) and \( b\). Start by convincing yourself that the two angles at C are indeed \( \alpha\) and \( \beta\), as marked. Now all that is required is to express \( \theta\) in terms of \( \alpha\) and \( \beta\).
Since the mid-point of the rod must be vertically below C, we must have AN = MB. That is:
\( b \sin \alpha = a \sin \beta\).
By the Sine Rule, \(\frac{b}{a} = \frac{\sin B}{\sin A}\), so that \(\sin \alpha \sin B = \sin \beta \sin A\)
But \(A = 90\)° \(- \alpha + \theta\) and \( B = 90\)° \(-\beta - \theta\), so
\(\sin \alpha = \cos(\beta - \theta) = \sin\beta\cos(\alpha + \theta) \),
which quickly yields \(\underline{\underline{\tan \theta = \frac{1}{2}(\cot \alpha - \cot \beta)}}\). In our particular example, this is \(\tan \theta = \frac{1}{2}(\sqrt{3} - 1), \underline{\underline{\theta = }}\)20.1°. If you wish, you could work out the forces at A and B in terms of the weight of the rod.
I have drawn above the three forces on the rod. The forces at the ends of the rod each make an angle \(\lambda\) to the normal to the surface, where \(\tan \lambda = \mu\), and the three coplanar forces, being in static equilibrium, are concurrent at a point. I have also introduced the angle \(\phi\), given by \(\cos \phi = l/a\). All we have to do is to find \(\theta\) in terms of \(\lambda\) and \(\phi\) - that is to say, in terms of \(\mu\) and \(l/a\)
Fortunately I found the following formula for a triangle in an old geometry book:
\(\cot \gamma = \frac{1}{2}(\cos \alpha - \cot \beta)\)
I’ll leave you to see if you can derive it. The book actually gave a formula for a more general case in which the base of the triangle isn’t divided equally. For the case
the formula is \( (1+x) \cot \gamma = \cot \alpha - x \cot \beta\).
You can use this in various problems in geometric optics, where you are trying to find relations between object distance, image distance and radius of curvature or focal length. However, for this problem, we need only the simpler formula, where the base of the triangle is equally divided.
On applying the simpler formula to our present problem we obtain
\( \underline{\underline{\tan \theta = \frac{1}{2}[\cot ( \phi - \lambda) - \cot( \phi + \lambda)]}},\)
and the problem is solved.
Below, I illustrate some examples. Going from left to right we have short \(l/a=0.2\), medium \(l/a=0.4\), long \(l/a= 0.6\) rod. Going from top to bottom we have a slippery \( \mu = 0.5\), a medium \(/mu = 1.0\), and a sticky \(/mu = 1.5\) surface.
Inside each drawing, I tabulate
\(l/a\)
\(/mu\)
\(\theta\)°
The long \((l / a =0.6)\) rod will rest vertically for any \(/mu > 1.33\). But, while it will not slip, the equilibrium is no longer stable, and the rod will tip after an infinitesimal counterclockwise displacement.
