11.6: Поля за законом Гауса
10.6.1 Закон Гауса
Блоха підрозділу 10.3.2 мала довгу і прославлену наукову кар'єру, і ми зараз збираємося підібрати її історію, де ми зупинилися. Ця блоха, назва якої Гаусс 9, вивела рівнянняE_\perp=2\pi k\sigma для електричного поля, дуже близького до зарядженої поверхні з щільністю заряду\sigma. Далі ми опишемо два вдосконалення, які вона збирається зробити в цьому рівнянні.
По-перше, вона розуміє, що рівняння не настільки корисне, як могло б бути, оскільки воно дає лише частину поля завдяки поверхні. Якщо інші заряди знаходяться поруч, то їх поля додадуть до цього поля як вектори, і рівняння не буде істинним, якщо ми не будемо ретельно віднімати поле з інших зарядів. Це особливо проблематично для неї, тому що планета, на якій вона живе, відома з незрозумілих причин як планета Flatcat, сама по собі електрично заряджена, і всі блохи - єдине, що утримує їх від спливу в космічний простір, це те, що вони негативно заряджені, в той час як Flatcat несе позитивний заряд, тому вони електрично притягуються до нього. Коли Гаусс знайшов оригінальну версію свого рівняння, вона хотіла продемонструвати його своїм скептично налаштованим колегам в лабораторії, використовуючи лічильники електричного поля та заряджені шматки металевої фольги. Навіть якщо вона налаштувала вимірювання за допомогою пульта дистанційного керування, щоб її заряд на власному тілі був би занадто далеко, щоб мати якийсь ефект, вони будуть порушені навколишнім полем планети Flatcat. Нарешті, однак, вона зрозуміла, що може покращити своє рівняння, переписавши його наступним чином:
Найскладніше тут полягає в тому, що «назовні» означає іншу річ, залежно від того, на якій стороні фольги ми знаходимося. З лівого боку «назовні» означає вліво, в той час як з правого боку «назовні» - справа. Позитивно заряджений шматок металевої фольги має поле, яке вказує вліво на лівій стороні, а праворуч на правій стороні, тому два внески обидва2\pi k\sigma позитивні, і ми отримуємо4\pi k\sigma. З іншого боку, припустимо, що є поле, створене іншими зарядами, а не зарядженою фольгою, що трапляється вказувати праворуч. З правого боку це зовнішньо створене поле знаходиться в тому ж напрямку, що і поле фольги, але з лівого боку воно зменшує силу лівого поля, створеного фольгою. Збільшення в одному семестрі рівняння врівноважує зменшення в іншому члені. Ця нова версія рівняння, таким чином, є точно правильною незалежно від того, які зовнішні породжені поля присутні!
Її наступне нововведення починається з множення рівняння з обох сторін на площу однієї сторони фольги:A
деq знаходиться заряд фольги. Причина цієї модифікації полягає в тому, що тепер вона може зробити все це більш привабливим, визначивши новий вектор, вектор області A.
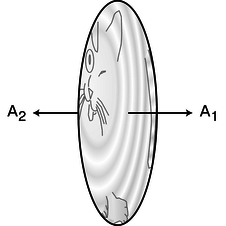
a / The area vector is defined to be perpendicular to the surface, in the outward direction. Its magnitude tells how much the area is.
As shown in figure a, she defines an area vector for side 1 which has magnitude A and points outward from side 1, and an area vector for side 2 which has the same magnitude and points outward from that side, which is in the opposite direction. The dot product of two vectors, \mathbf{u}\cdot\mathbf{v}, can be interpreted as u_{parallel\ to\ v}|\mathbf{v}|, and she can therefore rewrite her equation as
The quantity on the left side of this equation is called the flux through the surface, written \Phi.

b / Gauss contemplates a map of the known world.
Gauss now writes a grant proposal to her favorite funding agency, the BSGS (Blood-Suckers' Geological Survey), and it is quickly approved. Her audacious plan is to send out exploring teams to chart the electric fields of the whole planet of Flatcat, and thereby determine the total electric charge of the planet. The fleas' world is commonly assumed to be a flat disk, and its size is known to be finite, since the sun passes behind it at sunset and comes back around on the other side at dawn. The most daring part of the plan is that it requires surveying not just the known side of the planet but the uncharted Far Side as well. No flea has ever actually gone around the edge and returned to tell the tale, but Gauss assures them that they won't fall off --- their negatively charged bodies will be attracted to the disk no matter which side they are on.
Of course it is possible that the electric charge of planet Flatcat is not perfectly uniform, but that isn't a problem. As discussed in subsection 10.3.2, as long as one is very close to the surface, the field only depends on the local charge density. In fact, a side-benefit of Gauss's program of exploration is that any such local irregularities will be mapped out. But what the newspapers find exciting is the idea that once all the teams get back from their voyages and tabulate their data, the total charge of the planet will have been determined for the first time. Each surveying team is assigned to visit a certain list of republics, duchies, city-states, and so on. They are to record each territory's electric field vector, as well as its area. Because the electric field may be nonuniform, the final equation for determining the planet's electric charge will have many terms, not just one for each side of the planet:
Gauss herself leads one of the expeditions, which heads due east, toward the distant Tail Kingdom, known only from fables and the occasional account from a caravan of traders. A strange thing happens, however. Gauss embarks from her college town in the wetlands of the Tongue Republic, travels straight east, passes right through the Tail Kingdom, and one day finds herself right back at home, all without ever seeing the edge of the world! What can have happened? All at once she realizes that the world isn't flat.
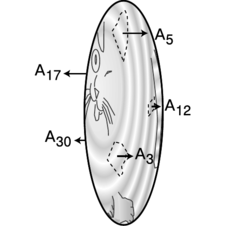
c / Each part of the surface has its own area vector. Note the differences in lengths of the vectors, corresponding to the unequal areas.
Now what? The surveying teams all return, the data are tabulated, and the result for the total charge of Flatcat is (1/4\pi k)\sum \mathbf{E}_j\cdot\mathbf{A}_j=37\ \text{nC} (units of nanocoulombs). But the equation was derived under the assumption that Flatcat was a disk. If Flatcat is really round, then the result may be completely wrong. Gauss and two of her grad students go to their favorite bar, and decide to keep on ordering Bloody Marys until they either solve their problems or forget them. One student suggests that perhaps Flatcat really is a disk, but the edges are rounded. Maybe the surveying teams really did flip over the edge at some point, but just didn't realize it. Under this assumption, the original equation will be approximately valid, and 37 nC really is the total charge of Flatcat.

d / An area vector can be defined for a sufficiently small part of a curved surface.
A second student, named Newton, suggests that they take seriously the possibility that Flatcat is a sphere. In this scenario, their planet's surface is really curved, but the surveying teams just didn't notice the curvature, since they were close to the surface, and the surface was so big compared to them. They divided up the surface into a patchwork, and each patch was fairly small compared to the whole planet, so each patch was nearly flat. Since the patch is nearly flat, it makes sense to define an area vector that is perpendicular to it. In general, this is how we define the direction of an area vector, as shown in figure d. This only works if the areas are small. For instance, there would be no way to define an area vector for an entire sphere, since “outward” is in more than one direction.
If Flatcat is a sphere, then the inside of the sphere must be vast, and there is no way of knowing exactly how the charge is arranged below the surface. However, the survey teams all found that the electric field was approximately perpendicular to the surface everywhere, and that its strength didn't change very much from one location to another. The simplest explanation is that the charge is all concentrated in one small lump at the center of the sphere. They have no way of knowing if this is really the case, but it's a hypothesis that allows them to see how much their 37 nC result would change if they assumed a different geometry. Making this assumption, Newton performs the following simple computation on a napkin. The field at the surface is related to the charge at the center by
where r is the radius of Flatcat. The flux is then
and since the \mathbf{E}_j and \mathbf{A}_j vectors are parallel, the dot product equals |\mathbf{E}_j||\mathbf{A}_j|, so
But the field strength is always the same, so we can take it outside the sum, giving
Not only have all the factors of r canceled out, but the result is the same as for a disk!
Everyone is pleasantly surprised by this apparent mathematical coincidence, but is it anything more than that? For instance, what if the charge wasn't concentrated at the center, but instead was evenly distributed throughout Flatcat's interior volume? Newton, however, is familiar with a result called the shell theorem (page 102), which states that the field of a uniformly charged sphere is the same as if all the charge had been concentrated at its center.10 We now have three different assumptions about the shape of Flatcat and the arrangement of the charges inside it, and all three lead to exactly the same mathematical result, \Phi = 4\pi kq_{total}. This is starting to look like more than a coincidence. In fact, there is a general mathematical theorem, called Gauss' theorem, which states the following:
For any region of space, the flux through the surface equals 4\pi kq_{in}, where q_{in} is the total charge in that region.
Don't memorize the factor of 4\pi in front --- you can rederive it any time you need to, by considering a spherical surface centered on a point charge.
Note that although region and its surface had a definite physical existence in our story --- they are the planet Flatcat and the surface of planet Flatcat --- Gauss' law is true for any region and surface we choose, and in general, the Gaussian surface has no direct physical significance. It's simply a computational tool.
Rather than proving Gauss' theorem and then presenting some examples and applications, it turns out to be easier to show some examples that demonstrate its salient properties. Having understood these properties, the proof becomes quite simple.
self-check:
Suppose we have a negative point charge, whose field points inward, and we pick a Gaussian surface which is a sphere centered on that charge. How does Gauss' theorem apply here?
(answer in the back of the PDF version of the book)
