1.2: Площина трикутної пластини

Означення: Медіана трикутника - це лінія від вершини до середини протилежної сторони.

Теорема I. Три медіани трикутника є одночасними (зустрічаються в одній унікальній точці) у точці, яка становить дві третини відстані від вершини до середини протилежної сторони.

Теорема II. Центр маси однорідної трикутної пластинки (або центроїда трикутника) знаходиться на зустрічі медіанів.

Доказ I можна зробити з хорошим векторним аргументом (рис. I.1):

$\bf{\text{A}}$$\bf{\text{B}}$Дозволяти , бути вектори$\text{OA}$ ,$\text{OB}$ . Потім$\bf{\text{A+B}}$ йде діагональ паралелограма, з якого$OA$ і$\text{OB}$ є дві сторони, і вектор положення точки$\text{C}_{1}$ is $\frac{1}{3}(\bf{\text{A+B}})$ .

Щоб отримати$\text{C}_{2}$, we see that

${\bf C}_2 = {\bf A} + \dfrac{2}{3}(\text{AM}_2) = {\bf A} + \dfrac{2}{3}({\bf M_2 - A}) = {\bf A} + \dfrac{2}{3}(\dfrac{1}{2}{\bf B- A}) = \dfrac{1}{3}( {\bf A+B})$

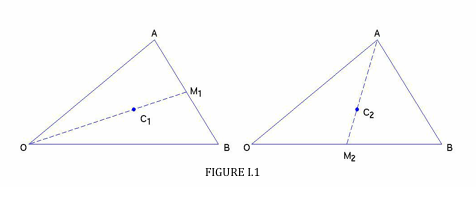
Таким чином, точки$\text{C}_{1}$ and $\text{C}_{2}$ are identical, and the same would be true for the third median, so Theorem I is proved.

Now consider an elemental slice as in Figure I.2. The centre of mass of the slice is at its mid-point. The same is true of any similar slices parallel to it. Therefore the centre of mass is on the locus of the mid-points - i.e. on a median. Similarly, it is on each of the other medians, and Theorem II is proved.

Для цього потрібна була лише певна векторна геометрія. Тепер перейдемо до деякого числення.