1.9: Теорема Гаусса

Точковий заряд\(Q\) is at the centre of a sphere of radius \(r\). Calculate the \(D\)-flux through the sphere. Easy. The magnitude of \(D\) at a distance \(a\) is \(Q/(4(\pi r^2)\) and the surface area of the sphere is 4\(\pi\)r². Therefore the flux is just \(Q\). Notice that this is independent of \(r\); if you double \(r\), the area is four times as great, but \(D\) is only a quarter of what it was, so the total flux remains the same. You will probably agree that if the charge is surrounded by a shape such as shown in Figure \(I\).8, which is made up of portions of spheres of different radii, the \(D\)-flux through the surface is still just \(Q\). And you can distort the surface as much as you like, or you may consider any surface to be made up of an infinite number of infinitesimal spherical caps, and you can put the charge anywhere you like inside the surface, or indeed you can put as many charges inside as you like – you haven’t changed the total normal component of the flux, which is still just \(Q\). This is Gauss’s theorem, which is a consequence of the inverse square nature of Coulomb’s law.

\(\text{FIGURE I.8}\)

Теорема Гаусса

Загальна нормальна складова\(D\) -потоку через будь-яку замкнуту поверхню дорівнює заряду, укладеному цією поверхнею.

Приклади

Довгий стрижень несе заряд\(\lambda\) per unit length. Construct around it a cylindrical surface of radius \(r\) and length \(l\). The charge enclosed is \(l\lambda\), and the field is directed radially outwards, passing only through the curved surface of the cylinder. The \(D\)-flux through the cylinder is \(l\lambda\) and the area of the curved surface is 2\(\pi rl\), so \(D = l\lambda /(2\pi rl)\) and hence \(E=\lambda / (2\pi\epsilon r)\).

\(\text{FIGURE I.9}\)

A flat plate carries a charge of \(\sigma\) per unit area. Construct around it a cylindrical surface of cross-sectional area \(A\). The charge enclosed by the cylinder is \(A\sigma\), so this is the \(D\)-flux through the cylinder. It all goes through the two ends of the cylinder, which have a total area 2\(A\), and therefore \(D\) = \(\sigma\)/2 and \(E = \sigma\)/(2\(\epsilon\)).

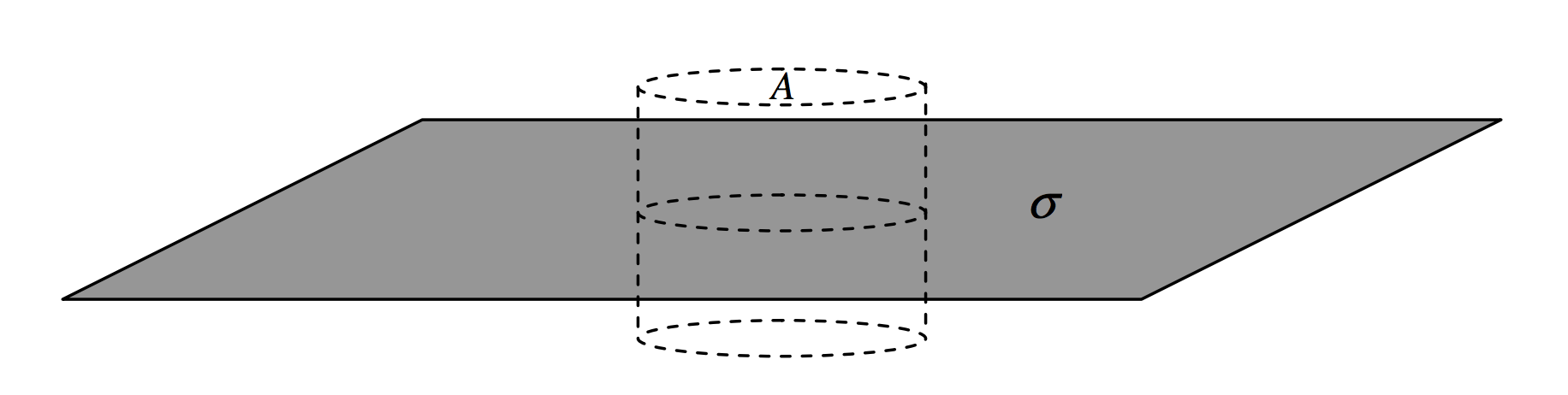
\(\text{FIGURE I.10}\)

Порожниста сферична оболонка радіусом a несе заряд\(Q\). Construct two gaussian spherical surfaces, one of radius less than \(a\) and the other of radius \(r > a\). The smaller of these two surfaces has no charge inside it; therefore the flux through it is zero, and so \(E\) is zero. The charge through the larger sphere is \(Q\) and is area is 4\(\pi r^2\). Therefore \(D=Q/(4\pi r^2)\text{ and }E=Q/(4\pi \epsilon r^2 )\). (It is worth going to Chapter 5 of Celestial Mechanics, subsection 5.4.8, to go through the calculus derivation, so that you can appreciate Gauss’s theorem all the more.)

Точковий заряд\(Q\) is in the middle of a cylinder of radius \(a\) and length \(2l\). Calculate the flux through the cylinder.

Нескінченний стрижень заряджається\(\lambda\) coulombs per unit length. It passes centrally through a spherical surface of radius\(a\). Calculate the flux through the spherical surface.

Ці завдання виконуються за допомогою обчислення в розділі 5.6 Небесної механіки, і надають хороші приклади того, як робити поверхневі інтеграли, і я рекомендую вам працювати над ними. Однак з теореми Гаусса очевидно, що відповіді є просто\(Q\) and \(2a\lambda\) respectively.

Точковий заряд\(Q\) is in the middle of a cube of side \(2a\). The flux through the cube is, by Gauss’s theorem, \(Q\), and the flux through one face is \(Q\)/6. I hope you enjoyed doing this by calculus in section 1.8.