1.8: флюс

Добутком напруженості електричного поля і площі є потік$\Phi_E$. Whereas $E$ is an intensive quantity, $\Phi_E$ is an extensive quantity. It dimensions are ML³T^-2Q^-1 and its SI units are N m² C^-1, although later on, after we have met the unit called the volt, we shall prefer to express $\Phi_E$ in V m.

Зі збільшенням ступенів витонченості флюс може бути визначено математично як:

$$\Phi_E = EA$$

$\text{FIGURE I.4}$: Потік, який перпендикулярний поверхні.

$$\Phi_E = EA \cos \theta = \textbf{E} \cdot \textbf{A}$$

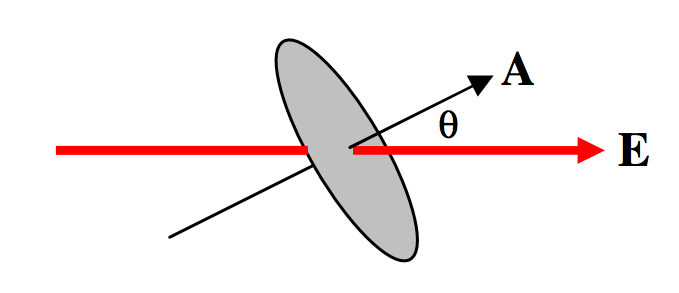
$\text{FIGURE I.5}$: Flow that is at an angle to the surface.

Зверніть увагу, що$\textbf{E}$ is a vector, but $\Phi_E$ is a scalar.

$$\Phi_E = \iint \textbf{E} \cdot d\textbf{A}$$

$\text{FIGURE I.6}$

Ми також можемо визначити$D$-flux by

$$\Phi_D=\iint \textbf{D}\cdot d\textbf{A}.$$

Розміри$\Phi_D$ are just $Q$ and the SI units are coulombs (C).

Приклад по порядку:

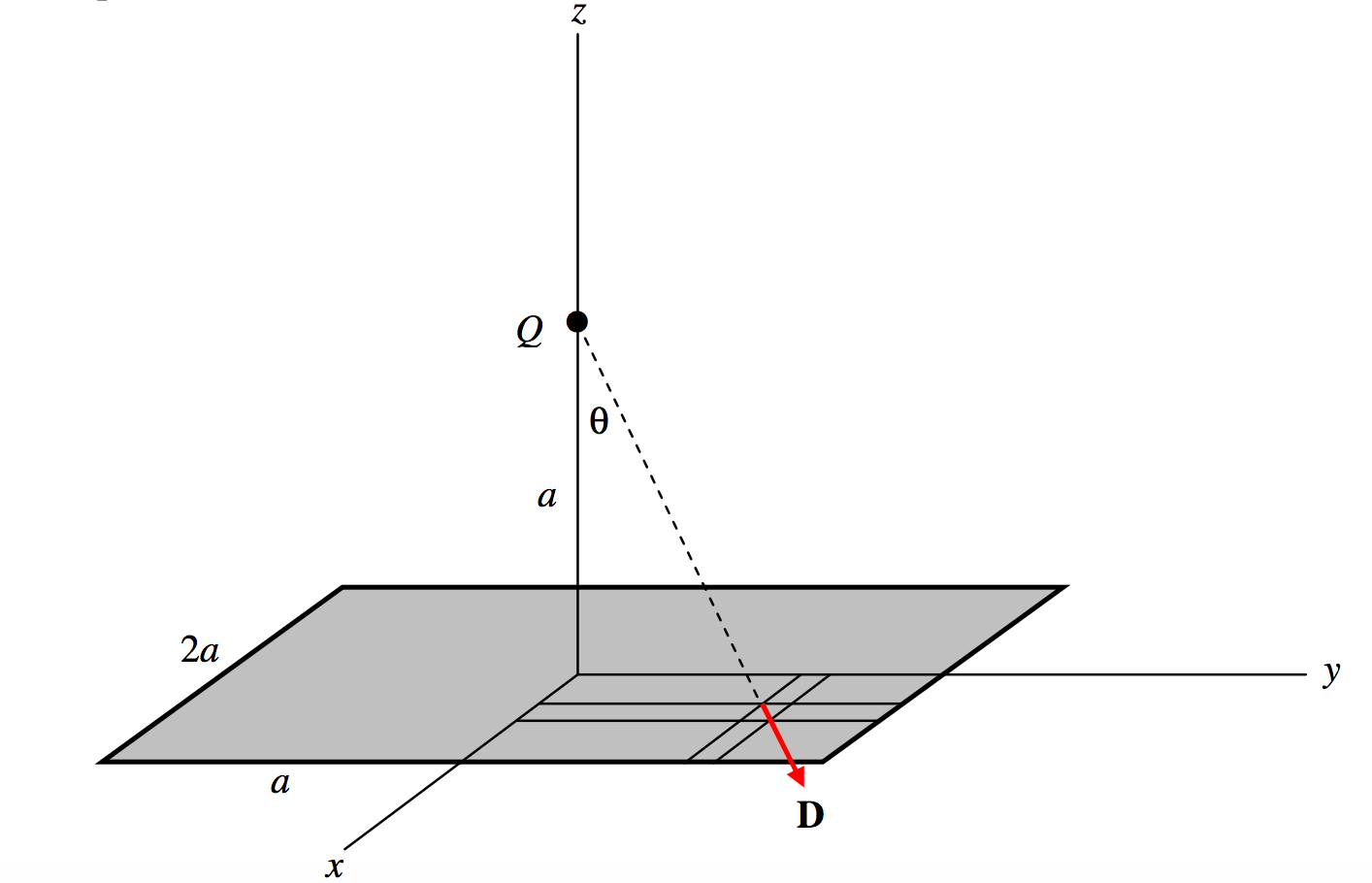
$\text{FIGURE I.7}$

Розглянемо квадрат сторони$2a$ in the xy-plane as shown. Suppose there is a positive charge $Q$ at a height $a$ on the $z$-axis. Calculate the total $D$-flux, $\Phi_D$ through the area.

Розглянемо стихійну область$dxdy$ at ($x,\, y ,\, 0$). Its distance from $Q$ is $(a^2+x^2+y^2)^{1/2}$ so the magnitude of the $D$-field there is $\frac{Q}{4\pi}\cdot \frac{1}{a^2+x^2+y^2}$. The scalar product of this with the area is $\frac{Q}{4\pi}\cdot \frac{1}{a^2+x^2+y^2}\cdot \cos \theta dxdy,\text{ and }\cos \theta = \frac{a}{(a^2+x^2+y^2)^{1/2}}$. The surface integral of $\textbf{D}$ over the whole area is

$$\label{1.8.1}\iint D\cdot dA=\frac{Qa}{\pi}\int_0^a \int_0^a \frac{dxdy}{(a^2+x^2+y^2)^{3/2}}.$$

Тепер все, що нам потрібно зробити, це хороший і легкий інтеграл. Нехай$x=\sqrt{a^2+y^2}\tan ψ$ and the inner integral $\int_0^a \frac{dx}{(a^2+x^2+y^2)^{3/2}}$ reduces, after some modest algebra, to $\frac{a}{(a^2+y^2)\sqrt{2a^2+y^2}}$. Thus we now have

$$\label{1.8.2}\iint D\cdot dA = \frac{Qa^2}{\pi}\int_0^a \frac{dy}{(a^2+y^2)\sqrt{2a^2+y^2}}.$$

З подальшою заміною$a^2+y^2=a^2 \sec \omega$ this reduces, after more careful algebra, to

$$\iint D\cdot dA=\frac{Q}{6}.\label{1.8.3}$$

Два додаткових приклади обчислення поверхневих інтегралів можна знайти в розділі 5.6 розділу «Небесна механіка» цих приміток. Вони мають справу з гравітаційними полями, але вони по суті такі ж, як електростатичний випадок; просто замінити$Q$ for m and -1/(4$\pi \epsilon$) for $G$.

Я закликаю читачів насправді пройти через біль і алгебру та тригонометрію цих трьох прикладів, щоб вони могли оцінити тим більше, у наступному розділі, силу теореми Гаусса.