1.9: Вимірювання кутів
Вимірювання кутів за допомогою транспортирів та постулат додавання кута.
Кути
Кут утворюється, коли два промені мають однакову кінцеву точку. Вершина є загальною кінцевою точкою двох променів, які утворюють кут. Сторони - це два промені, які утворюють кут.
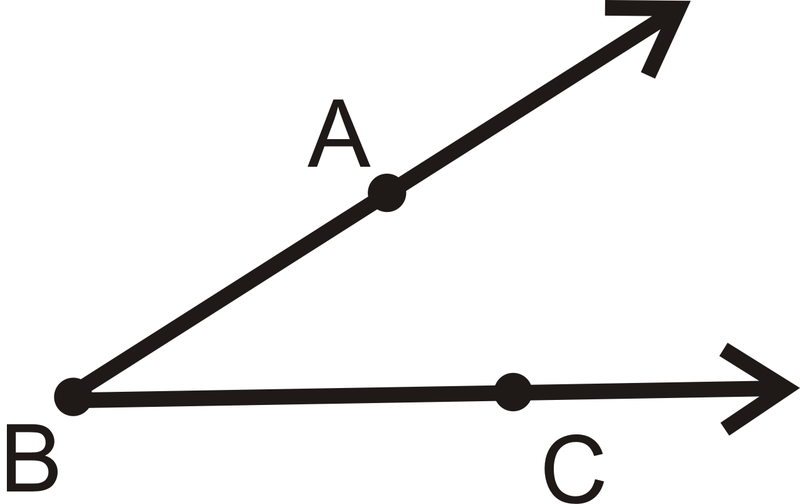 Малюнок1.9.1
Малюнок1.9.1| Позначте це | Скажи це |
|---|---|
| ∠ABC | КутABC |
| ∠CBA | КутCBA |
Вершина є,B а сторони -→BA і→BC. Завжди використовуйте три літери, щоб назвати кут,∠ SIDE-VERTEX-SIDE.
Кути вимірюються чимось, званим транспортиром. Транспортир - це вимірювальний прилад, який вимірює, наскільки «відкритий» кут. Кути вимірюються в градусах і маркуються∘ символом. Наразі кути завжди позитивні.
 Малюнок1.9.2
Малюнок1.9.2Є два набори вимірювань, один починається зліва, а інший з правого боку транспортира. Обидва йдуть навколо від0∘ до180∘. При вимірюванні кутів можна вирівняти одну сторону з0∘, і подивитися, де інша сторона потрапляє на транспортир. Вершина лінії вгору посередині лінії низу.
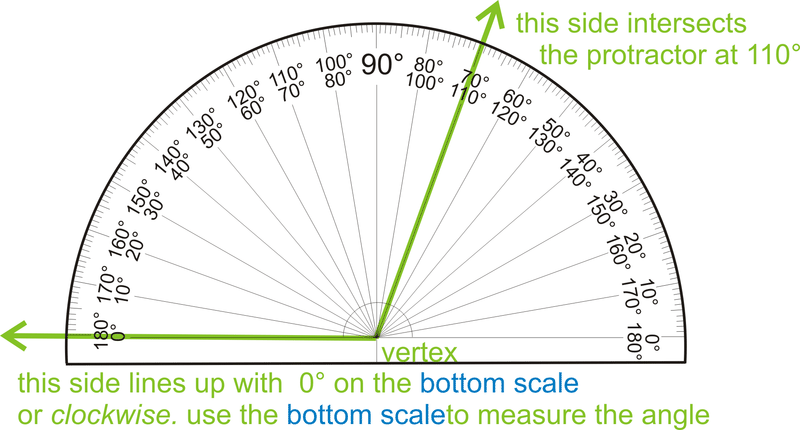 Малюнок1.9.3
Малюнок1.9.3Зверніть увагу, що якщо ви не вирівнюєте одну сторону з0∘, міра кута буде різницею градусів, де сторони кута перетинаються з транспортиром.
Іноді вам захочеться намалювати кут, який є певною кількістю градусів. Виконайте наведені нижче дії, щоб намалювати50∘ кут за допомогою транспортира:
- Почніть з малювання горизонтальної лінії по всій сторінці, довжиною 2 дюйма.
 Малюнок1.9.4
Малюнок1.9.4- Помістіть кінцеву точку в лівій частині лінії.
- Помістіть транспортир на цю точку, таким чином, щоб лінія проходила через0∘ мітку на транспортирі, а кінцева точка виявилася в центрі. Відзначте50∘ на відповідній шкалі.
 \(\Індекс сторінки малюнка {5}\)
\(\Індекс сторінки малюнка {5}\)- Зніміть транспортир і з'єднайте вершину і50∘ mark.
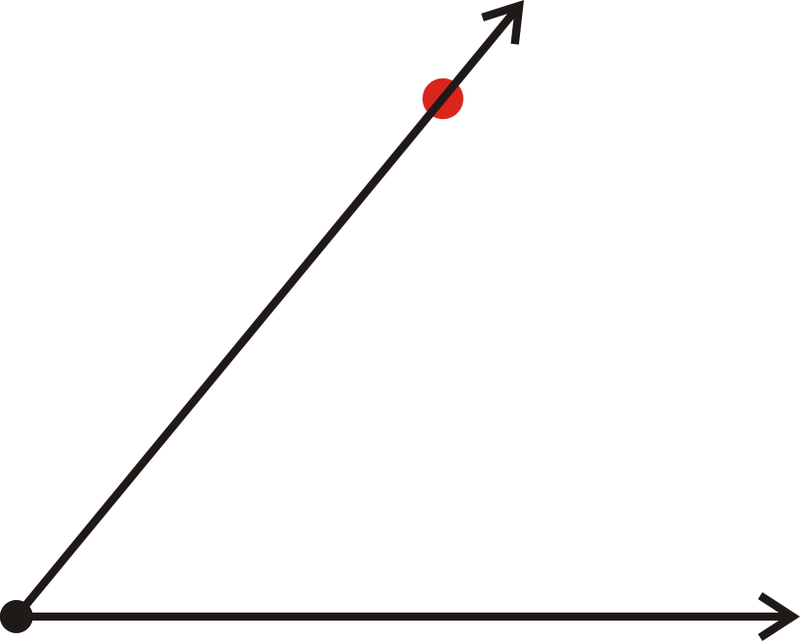 Figure 1.9.6
Figure 1.9.6This process can be used to draw any angle between 0∘ and 180∘.
When two smaller angles form to make a larger angle, the sum of the measures of the smaller angles will equal the measure of the larger angle. This is called the Angle Addition Postulate. So, if B is on the interior of ∠ADC, then
∠ADC=m∠ADB+∠BDC
 Figure 1.9.7
Figure 1.9.7Example 1.9.1
How many angles are in the picture below? Label each one.
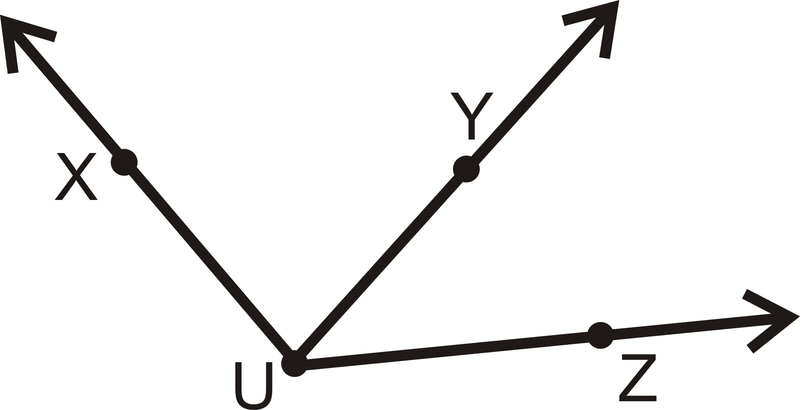 Figure 1.9.8
Figure 1.9.8Solution
There are three angles with vertex U. It might be easier to see them all if we separate them.
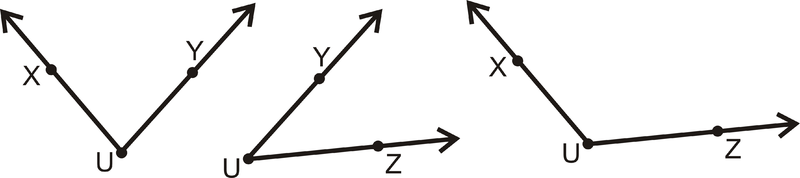 Figure 1.9.9
Figure 1.9.9So, the three angles can be labeled, ( ∠XUY or ∠YUX), ∠YUZ (or ∠ZUY), and ∠XUZ (or ∠ZUX).
Example 1.9.2
Measure the three angles from Example 1, using a protractor.
 Figure 1.9.10
Figure 1.9.10Solution
Just like in Example 1, it might be easier to measure these three angles if we separate them.
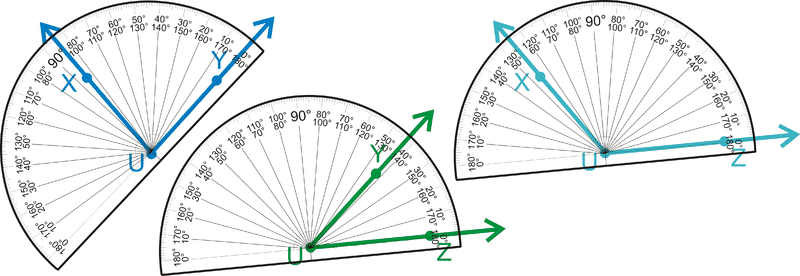 Figure 1.9.11
Figure 1.9.11With measurement, we put an m in front of the ∠ sign to indicate measure. So, m∠XUY=84∘, m∠YUZ=42∘ and m∠XUZ=126∘.
Example 1.9.3
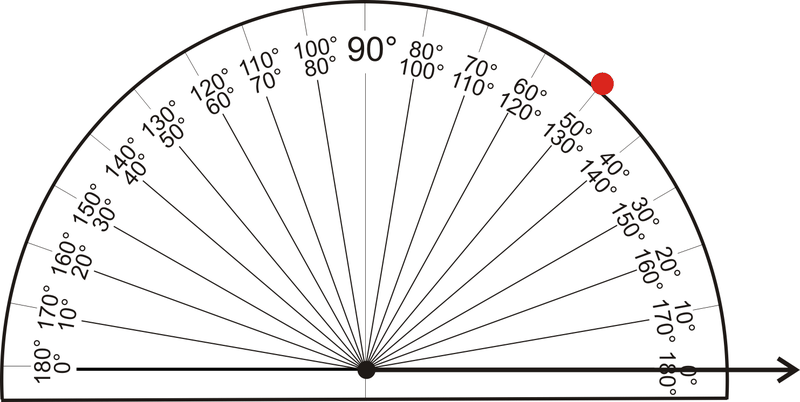
What is the measure of the angle shown below?
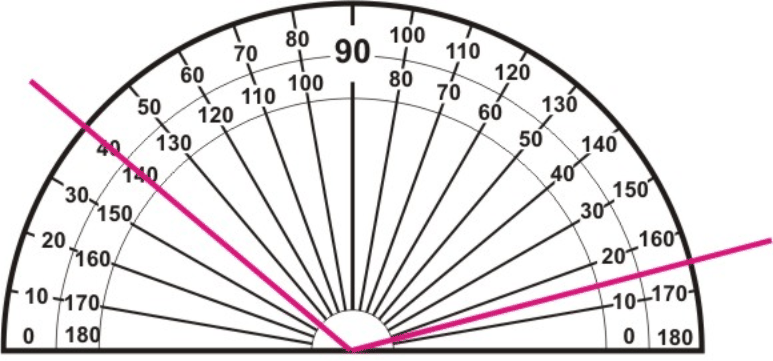 Figure 1.9.12
Figure 1.9.12Solution
This angle is not lined up with 0∘, so use subtraction to find its measure. It does not matter which scale you use, as long as you are consistent.
Inner scale: 140∘−15∘=125∘
Outer scale: 165∘−40∘=125∘
Example 1.9.4
Use a protractor to measure ∠RST below.
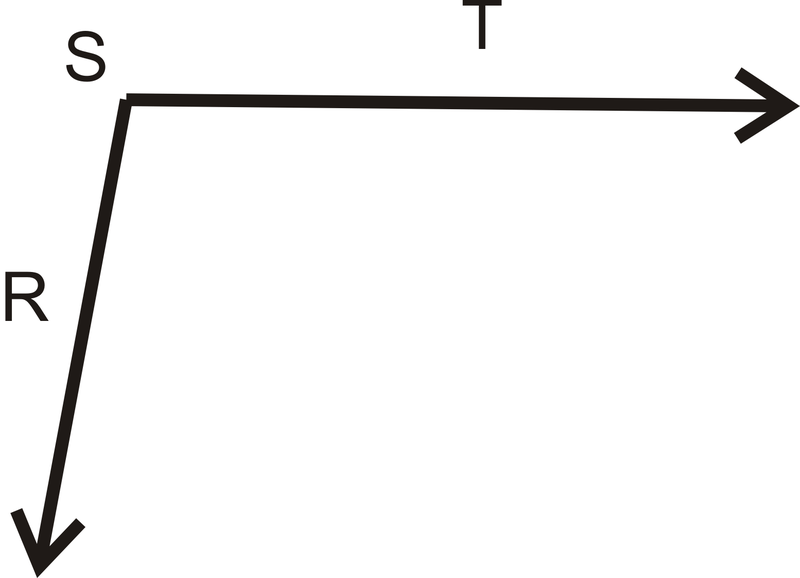 Figure 1.9.13
Figure 1.9.13Solution
Lining up one side with 0∘ on the protractor, the other side hits 100∘.
Example 1.9.5
What is m∠QRT in the diagram below?
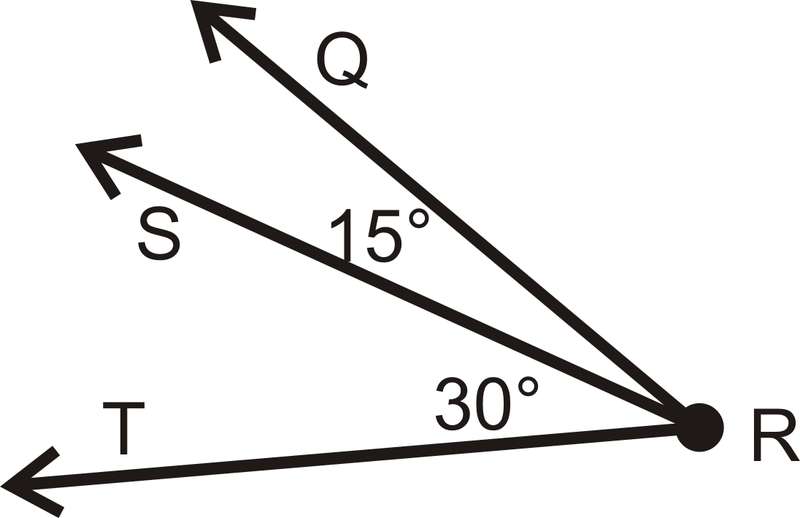 Figure 1.9.14
Figure 1.9.14Solution
Using the Angle Addition Postulate, m∠QRT=15∘+30∘=45∘.
Review
1. What is m∠LMN if m∠LMO=85∘ and m∠NMO=53∘?
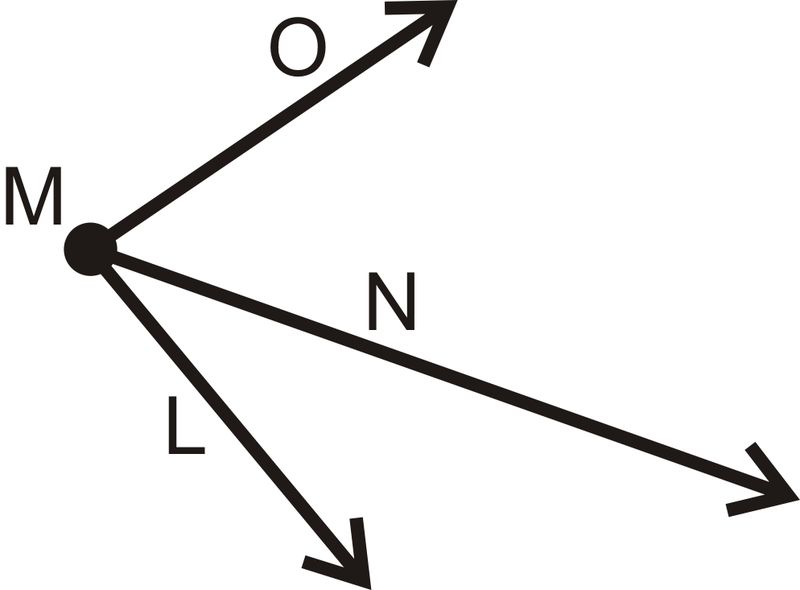 Figure 1.9.15
Figure 1.9.152. If m∠ABD=100∘, find x.
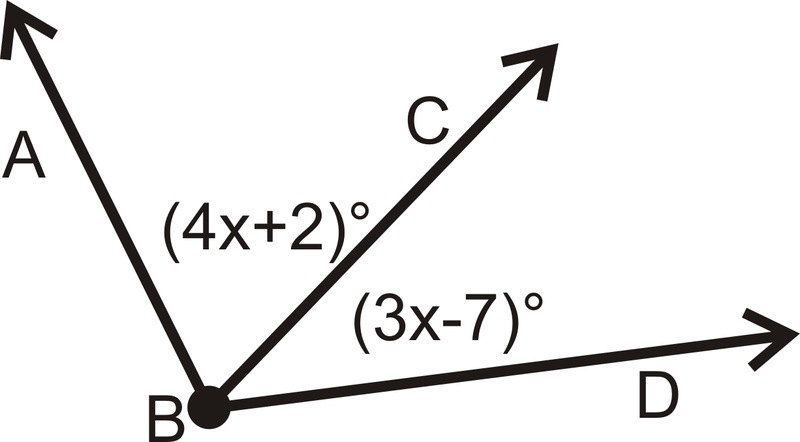 Figure 1.9.16
Figure 1.9.16For questions 3-6, determine if the statement is true or false.
- For an angle ∠ABC,C is the vertex.
- For an angle ∠ABC, ¯AB and ¯BC are the sides.
- The m in front of m∠ABC means measure.
- The Angle Addition Postulate says that an angle is equal to the sum of the smaller angles around it.
For 7-12, draw the angle with the given degree, using a protractor and a ruler.
- 55∘
- 92∘
- 178∘
- 5∘
- 120∘
- 73∘
For 13-16, use a protractor to determine the measure of each angle.
-
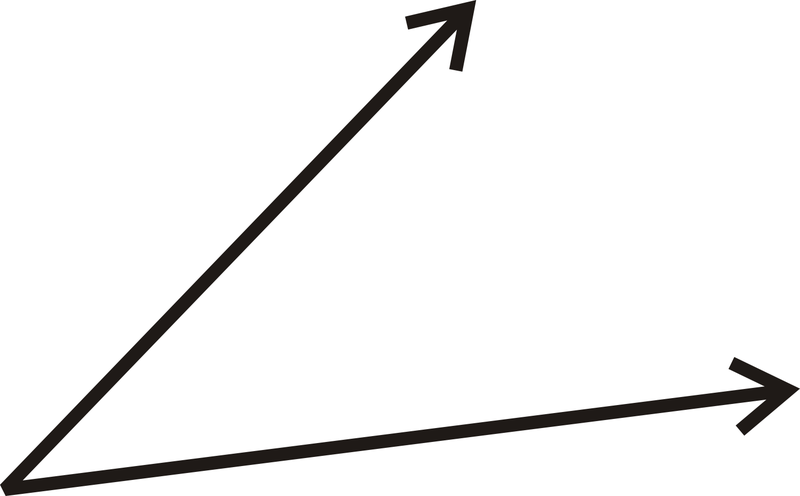 Figure 1.9.17
Figure 1.9.17 -
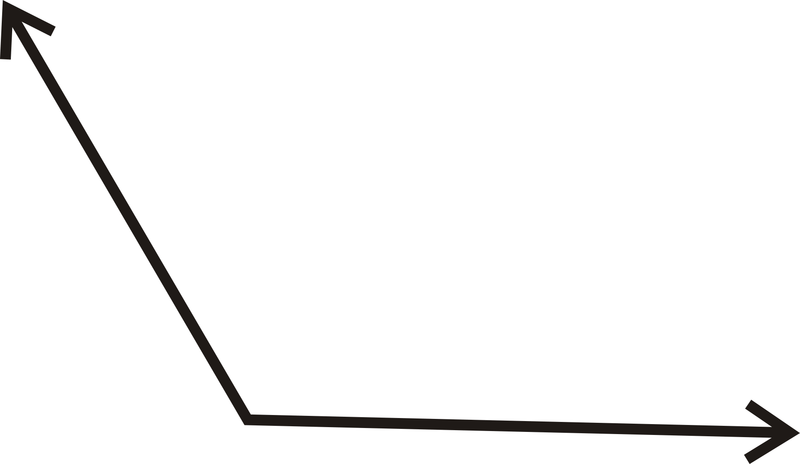 Figure 1.9.18
Figure 1.9.18 -
 Figure 1.9.19
Figure 1.9.19 -
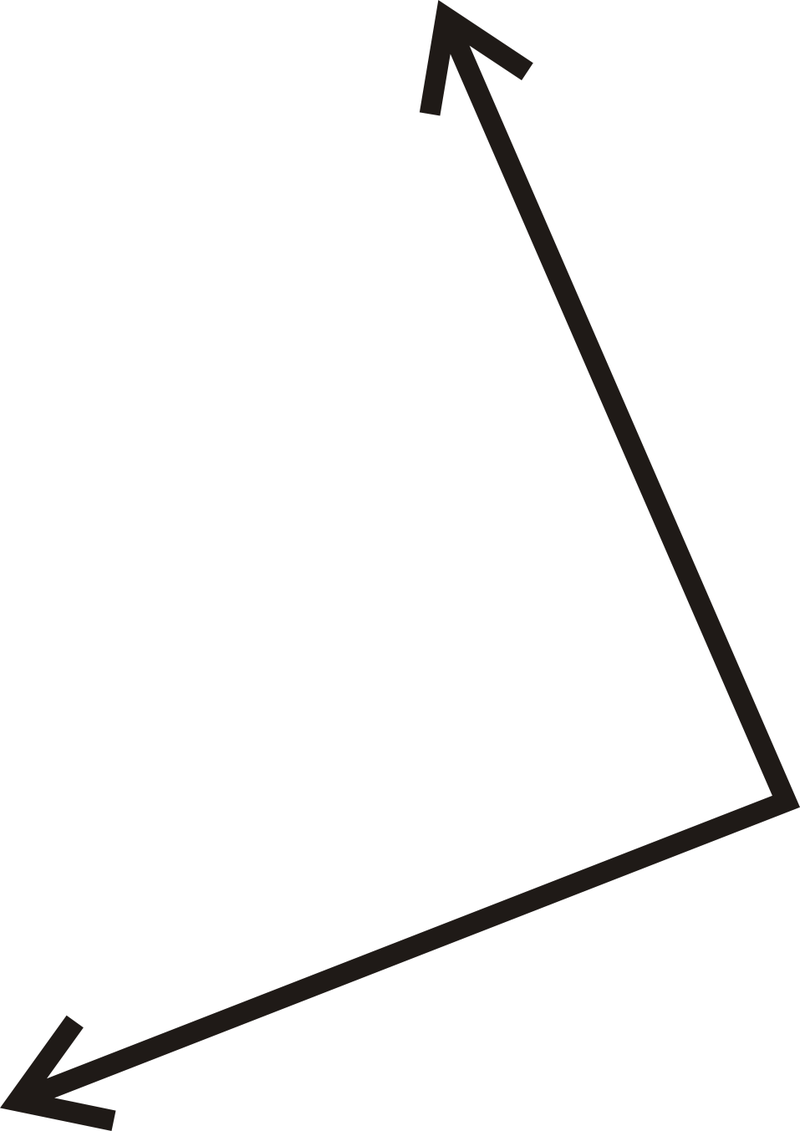 Figure 1.9.20
Figure 1.9.20
Solve for x.
- m∠ADC=56∘
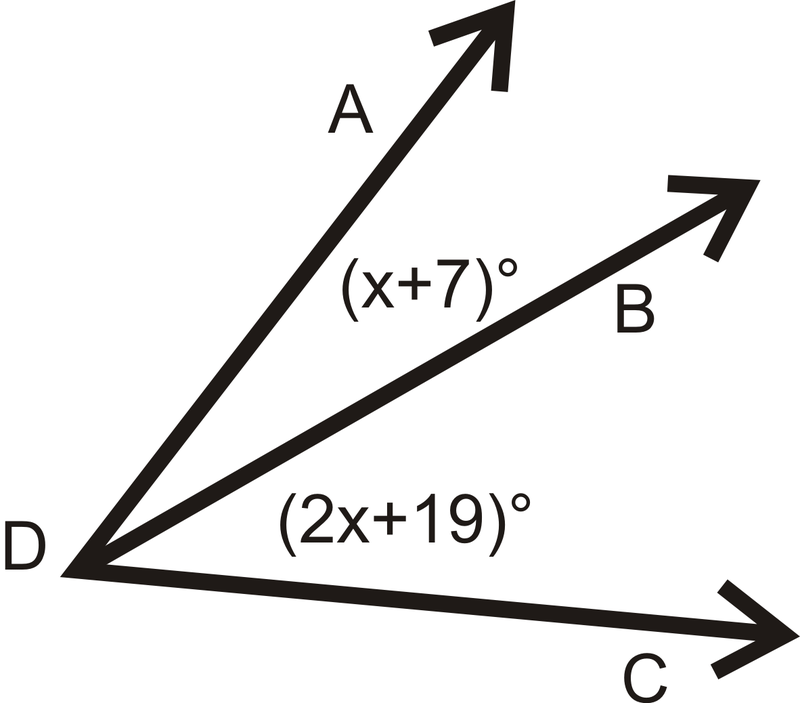 Figure 1.9.21
Figure 1.9.21Review (Answers)
To see the Review answers, open this PDF file and look for section 1.5.
Vocabulary
| Term | Definition |
|---|---|
| Angle | A geometric figure formed by two rays that connect at a single point or vertex. |
| Protractor | A protractor is a tool used to measure an angle in terms of degrees. |
| Vertex | A vertex is a point of intersection of the lines or rays that form an angle. |
Additional Resource
Interactive Element
Video: Angle Basics
Activities: Angle Measurement Discussion Questions
Study Aids: Angles Study Guide
Practice: Measuring Angles
Real World: Angle Measurement